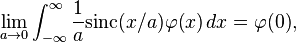Sinc Function - Relationship To The Dirac Delta Distribution

Relationship To The Dirac Delta Distribution

The normalized sinc function can be used as a nascent delta function, meaning that the following weak limit holds:

This is not an ordinary limit, since the left side does not converge. Rather, it means that

\lim_{a\rightarrow 0}\int_{-\infty}^\infty \frac{1}{a}\textrm{sinc}(x/a)\varphi(x)\,dx = \varphi(0),

for any smooth function with compact support.

In the above expression, as a approaches zero, the number of oscillations per unit length of the sinc function approaches infinity. Nevertheless, the expression always oscillates inside an envelope of ±1/(π a x), and approaches zero for any nonzero value of x. This complicates the informal picture of δ(x) as being zero for all x except at the point x = 0 and illustrates the problem of thinking of the delta function as a function rather than as a distribution. A similar situation is found in the Gibbs phenomenon.

Read more about this topic:  Sinc Function

Famous quotes containing the words relationship to the, relationship to, relationship and/or distribution:

    “Whatever may be our just grievances in the southern states, it is fitting that we acknowledge that, considering their poverty and past relationship to the Negro race, they have done remarkably well for the cause of education among us. That the whole South should commit itself to the principle that the colored people have a right to be educated is an immense acquisition to the cause of popular education.”
    —Fannie Barrier Williams (1855–1944)

    “Film music should have the same relationship to the film drama that somebody’s piano playing in my living room has to the book I am reading.”
    —Igor Stravinsky (1882–1971)

    “Every relationship that does not raise us up pulls us down, and vice versa; this is why men usually sink down somewhat when they take wives while women are usually somewhat raised up. Overly spiritual men require marriage every bit as much as they resist it as bitter medicine.”
    —Friedrich Nietzsche (1844–1900)

    “The man who pretends that the distribution of income in this country reflects the distribution of ability or character is an ignoramus. The man who says that it could by any possible political device be made to do so is an unpractical visionary. But the man who says that it ought to do so is something worse than an ignoramous and more disastrous than a visionary: he is, in the profoundest Scriptural sense of the word, a fool.”
    —George Bernard Shaw (1856–1950)