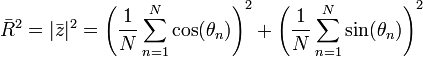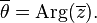Von Mises Distribution - Distribution of The Mean

Distribution of The Mean

The distribution of the sample mean for the von Mises distribution is given by:

P(\bar{R},\bar{\theta})\,d\bar{R}\,d\bar{\theta}=\frac{1}{ (2\pi I_0(k))^N}\int_\Gamma \prod_{n=1}^N \left( e^{\kappa\cos(\theta_n-\mu)} d\theta_n\right) = \frac{e^{\kappa N\bar{R}\cos(\bar{\theta}-\mu)}}{I_0(\kappa)^N}\left(\frac{1}{(2\pi)^N}\int_\Gamma \prod_{n=1}^N d\theta_n\right)

where N is the number of measurements and consists of intervals of in the variables, subject to the constraint that and are constant, where is the mean resultant:

\bar{R}^2=|\bar{z}|^2= \left(\frac{1}{N}\sum_{n=1}^N \cos(\theta_n) \right)^2 + \left(\frac{1}{N}\sum_{n=1}^N \sin(\theta_n) \right)^2

and is the mean angle:

\overline{\theta}=\mathrm{Arg}(\overline{z}). \,

Note that product term in parentheses is just the distribution of the mean for a circular uniform distribution.

Read more about this topic:  Von Mises Distribution

Famous quotes containing the words distribution of and/or distribution:

    “The man who pretends that the distribution of income in this country reflects the distribution of ability or character is an ignoramus. The man who says that it could by any possible political device be made to do so is an unpractical visionary. But the man who says that it ought to do so is something worse than an ignoramous and more disastrous than a visionary: he is, in the profoundest Scriptural sense of the word, a fool.”
    —George Bernard Shaw (1856–1950)

    “The question for the country now is how to secure a more equal distribution of property among the people. There can be no republican institutions with vast masses of property permanently in a few hands, and large masses of voters without property.... Let no man get by inheritance, or by will, more than will produce at four per cent interest an income ... of fifteen thousand dollars] per year, or an estate of five hundred thousand dollars.”
    —Rutherford Birchard Hayes (1822–1893)