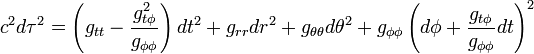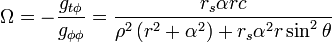Frame-dragging - Mathematical Derivation of Frame-dragging | Mathematical Derivation Frame-dragging

Mathematical Derivation of Frame-dragging

Frame-dragging may be illustrated most readily using the Kerr metric, which describes the geometry of spacetime in the vicinity of a mass M rotating with angular momentum J

$c^{2} d\tau^{2} = \left( 1 - \frac{r_{s} r}{\rho^{2}} \right) c^{2} dt^{2} - \frac{\rho^{2}}{\Lambda^{2}} dr^{2} - \rho^{2} d\theta^{2}$

$- \left( r^{2} + \alpha^{2} + \frac{r_{s} r \alpha^{2}}{\rho^{2}} \sin^{2} \theta \right) \sin^{2} \theta \ d\phi^{2} + \frac{2r_{s} r\alpha c \sin^{2} \theta }{\rho^{2}} d\phi dt$

where r_s is the Schwarzschild radius

$r_{s} = \frac{2GM}{c^{2}}$

and where the following shorthand variables have been introduced for brevity

$\alpha = \frac{J}{Mc}$

$\rho^{2} = r^{2} + \alpha^{2} \cos^{2} \theta\,\!$

$\Lambda^{2} = r^{2} - r_{s} r + \alpha^{2}\,\!$

In the non-relativistic limit where M (or, equivalently, r_s) goes to zero, the Kerr metric becomes the orthogonal metric for the oblate spheroidal coordinates

$c^{2} d\tau^{2} = c^{2} dt^{2} - \frac{\rho^{2}}{r^{2} + \alpha^{2}} dr^{2} - \rho^{2} d\theta^{2} - \left( r^{2} + \alpha^{2} \right) \sin^{2}\theta d\phi^{2}$

We may re-write the Kerr metric in the following form

$c^{2} d\tau^{2} = \left( g_{tt} - \frac{g_{t\phi}^{2}}{g_{\phi\phi}} \right) dt^{2} + g_{rr} dr^{2} + g_{\theta\theta} d\theta^{2} + g_{\phi\phi} \left( d\phi + \frac{g_{t\phi}}{g_{\phi\phi}} dt \right)^{2}$

This metric is equivalent to a co-rotating reference frame that is rotating with angular speed Ω that depends on both the radius r and the colatitude θ

$\Omega = -\frac{g_{t\phi}}{g_{\phi\phi}} = \frac{r_{s} \alpha r c}{\rho^{2} \left( r^{2} + \alpha^{2} \right) + r_{s} \alpha^{2} r \sin^{2}\theta}$

In the plane of the equator this simplifies to:

$\Omega = \frac{r_{s} \alpha c}{r^{3} + \alpha^{2} r + r_{s} \alpha^{2}}$

Thus, an inertial reference frame is entrained by the rotating central mass to participate in the latter's rotation; this is frame-dragging.

An extreme version of frame dragging occurs within the ergosphere of a rotating black hole. The Kerr metric has two surfaces on which it appears to be singular. The inner surface corresponds to a spherical event horizon similar to that observed in the Schwarzschild metric; this occurs at

$r_{inner} = \frac{r_{s} + \sqrt{r_{s}^{2} - 4\alpha^{2}}}{2}$

where the purely radial component g_rr of the metric goes to infinity. The outer surface is not a sphere, but an oblate spheroid that touches the inner surface at the poles of the rotation axis, where the colatitude θ equals 0 or π; its radius is defined by the formula

$r_{outer} = \frac{r_{s} + \sqrt{r_{s}^{2} - 4\alpha^{2} \cos^{2}\theta}}{2}$

where the purely temporal component g_tt of the metric changes sign from positive to negative. The space between these two surfaces is called the ergosphere. A moving particle experiences a positive proper time along its worldline, its path through spacetime. However, this is impossible within the ergosphere, where g_tt is negative, unless the particle is co-rotating with the interior mass M with an angular speed at least of Ω. However, as seen above, frame-dragging occurs about every rotating mass and at every radius r and colatitude θ, not only within the ergosphere.

Read more about this topic: Frame-dragging

Famous quotes containing the word mathematical:

“What he loved so much in the plant morphological structure of the tree was that given a fixed mathematical basis, the final evolution was so incalculable.”
—D.H. (David Herbert)