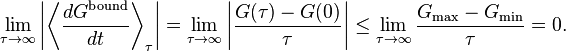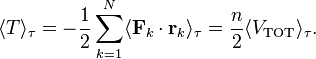Virial Theorem

The virial theorem states that, if, then

$2 \left\langle T \right\rangle_\tau = -\sum_{k=1}^N \left\langle \mathbf{F}_k \cdot \mathbf{r}_k \right\rangle_\tau.$

There are many reasons why the average of the time derivative might vanish, i.e., . One often-cited reason applies to stably bound systems, i.e., systems that hang together forever and whose parameters are finite. In that case, velocities and coordinates of the particles of the system have upper and lower limits so that the virial, Gbound, is bounded between two extremes, G_min and G_max, and the average goes to zero in the limit of very long times τ

$\lim_{\tau \rightarrow \infty} \left| \left\langle \frac{dG^{\mathrm{bound}}}{dt} \right\rangle_\tau \right| = \lim_{\tau \rightarrow \infty} \left| \frac{G(\tau) - G(0)}{\tau} \right| \le \lim_{\tau \rightarrow \infty} \frac{G_\max - G_\min}{\tau} = 0.$

Even if the average of the time derivative of G is only approximately zero, the virial theorem holds to the same degree of approximation.

For power-law forces with an exponent n, the general equation holds

$\langle T \rangle_\tau = -\frac{1}{2} \sum_{k=1}^N \langle \mathbf{F}_k \cdot \mathbf{r}_k \rangle_\tau = \frac{n}{2} \langle V_\text{TOT} \rangle_\tau.$

For gravitational attraction, n equals −1 and the average kinetic energy equals half of the average negative potential energy

$\langle T \rangle_\tau = -\frac{1}{2} \langle V_\text{TOT} \rangle_\tau.$

This general result is useful for complex gravitating systems such as solar systems or galaxies.

A simple application of the virial theorem concerns galaxy clusters. If a region of space is unusually full of galaxies, it is safe to assume that they have been together for a long time, and the virial theorem can be applied. Doppler measurements give lower bounds for their relative velocities, and the virial theorem gives a lower bound for the total mass of the cluster, including any dark matter.

The averaging need not be taken over time; an ensemble average can also be taken, with equivalent results.

Although derived for classical mechanics, the virial theorem also holds for quantum mechanics, which was proved by Fock (the quantum equivalent of the l.h.s. vanishes for energy eigenstates).

Read more about Virial Theorem: In Special Relativity, Generalizations, Inclusion of Electromagnetic Fields, In Astrophysics

Famous quotes containing the word theorem:

“To insure the adoration of a theorem for any length of time, faith is not enough, a police force is needed as well.”
—Albert Camus (1913–1960)