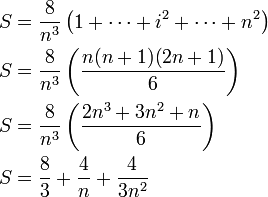Riemann Sum - Example

Example

Taking an example, the area under the curve of between 0 and 2 can be procedurally computed using Riemann's method.

The interval from 0 to 2 is firstly divided into n subintervals, each of which is given a width of ; these are the widths of the Riemann rectangles. Because the right Riemann sum is to be used, the sequence of x coordinates for the boxes will be . Therefore, the sequence of the heights of the boxes will be . It is an important fact that, and .

The area of each box will be and therefore the nth right Riemann sum will be: . Hence:

$\begin{align} S &= \frac{8}{n^3} \left(1 + \cdots + i^2 + \cdots + n^2\right)\\ S &= \frac{8}{n^3} \left(\frac{n(n+1)(2n+1)}{6}\right)\\ S &= \frac{8}{n^3} \left(\frac{2n^3+3n^2+n}{6}\right)\\ S &= \frac{8}{3} + \frac{4}{n} + \frac{4}{3n^2} \end{align}$

If the limit is viewed as, it can be concluded that the approximation approaches the actual value of the area under the curve as the number of boxes increases. Hence:

$\begin{align} S &= \lim_{n \rightarrow \infty}\left(\frac{8}{3} + \frac{4}{n} + \frac{4}{3n^2}\right)\\ S &= \frac{8}{3} \end{align}$

This method agrees with the definite integral as calculated in more mechanical ways:

Famous quotes containing the word example:

“Our intellect is not the most subtle, the most powerful, the most appropriate, instrument for revealing the truth. It is life that, little by little, example by example, permits us to see that what is most important to our heart, or to our mind, is learned not by reasoning but through other agencies. Then it is that the intellect, observing their superiority, abdicates its control to them upon reasoned grounds and agrees to become their collaborator and lackey.”
—Marcel Proust (1871–1922)