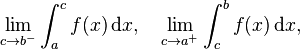Improper Integral

In calculus, an improper integral is the limit of a definite integral as an endpoint of the interval(s) of integration approaches either a specified real number or โˆž or โˆ’โˆž or, in some cases, as both endpoints approach limits.

Specifically, an improper integral is a limit of the form

or of the form

\lim_{c\to b^-} \int_a^cf(x)\, \mathrm{d}x,\quad \lim_{c\to a^+} \int_c^bf(x)\, \mathrm{d}x,

in which one takes a limit in one or the other (or sometimes both) endpoints (Apostol 1967, ยง10.23). Integrals are also improper if the integrand is undefined at an interior point of the domain of integration, or at multiple such points.

It is often necessary to use improper integrals in order to compute a value for integrals which may not exist in the conventional sense (as a Riemann integral, for instance) because of a singularity in the function, or an infinite endpoint of the domain of integration.

Read more about Improper Integral:  Examples, Convergence of The Integral, Types of Integrals, Improper Riemann Integrals and Lebesgue Integrals, Singularities, Cauchy Principal Value, Summability, Bibliography

Famous quotes containing the words improper and/or integral:

    “If the national security is involved, anything goes. There are no rules. There are people so lacking in roots about what is proper and what is improper that they don’t know there’s anything wrong in breaking into the headquarters of the opposition party.”
    —Helen Gahagan Douglas (1900–1980)

    “An island always pleases my imagination, even the smallest, as a small continent and integral portion of the globe. I have a fancy for building my hut on one. Even a bare, grassy isle, which I can see entirely over at a glance, has some undefined and mysterious charm for me.”
    —Henry David Thoreau (1817–1862)