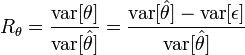A Comparison of Classical and Item Response Theories
Classical test theory (CTT) and IRT are largely concerned with the same problems but are different bodies of theory and entail different methods. Although the two paradigms are generally consistent and complementary, there are a number of points of difference:
- IRT makes stronger assumptions than CTT and in many cases provides correspondingly stronger findings; primarily, characterizations of error. Of course, these results only hold when the assumptions of the IRT models are actually met.
- Although CTT results have allowed important practical results, the model-based nature of IRT affords many advantages over analogous CTT findings.
- CTT test scoring procedures have the advantage of being simple to compute (and to explain) whereas IRT scoring generally requires relatively complex estimation procedures.
- IRT provides several improvements in scaling items and people. The specifics depend upon the IRT model, but most models scale the difficulty of items and the ability of people on the same metric. Thus the difficulty of an item and the ability of a person can be meaningfully compared.
- Another improvement provided by IRT is that the parameters of IRT models are generally not sample- or test-dependent whereas true-score is defined in CTT in the context of a specific test. Thus IRT provides significantly greater flexibility in situations where different samples or test forms are used. These IRT findings are foundational for computerized adaptive testing.
It is worth also mentioning some specific similarities between CTT and IRT which help to understand the correspondence between concepts. First, Lord showed that under the assumption that is normally distributed, discrimination in the 2PL model is approximately a monotonic function of the point-biserial correlation. In particular:
where is the point biserial correlation of item i. Thus, if the assumption holds, where there is a higher discrimination there will generally be a higher point-biserial correlation.
Another similarity is that while IRT provides for a standard error of each estimate and an information function, it is also possible to obtain an index for a test as a whole which is directly analogous to Cronbach's alpha, called the separation index. To do so, it is necessary to begin with a decomposition of an IRT estimate into a true location and error, analogous to decomposition of an observed score into a true score and error in CTT. Let
where is the true location, and is the error association with an estimate. Then is an estimate of the standard deviation of for person with a given weighted score and the separation index is obtained as follows
where the mean squared standard error of person estimate gives an estimate of the variance of the errors, across persons. The standard errors are normally produced as a by-product of the estimation process. The separation index is typically very close in value to Cronbach's alpha.
IRT is sometimes called strong true score theory or modern mental test theory because it is a more recent body of theory and makes more explicit the hypotheses that are implicit within CTT.
Read more about this topic: Item Response Theory
Famous quotes containing the words comparison, classical, item, response and/or theories:
“In comparison to the French Revolution, the American Revolution has come to seem a parochial and rather dull event. This, despite the fact that the American Revolution was successful—realizing the purposes of the revolutionaries and establishing a durable political regime—while the French Revolution was a resounding failure, devouring its own children and leading to an imperial despotism, followed by an eventual restoration of the monarchy.”
—Irving Kristol (b. 1920)
“Several classical sayings that one likes to repeat had quite a different meaning from the ones later times attributed to them.”
—Johann Wolfgang Von Goethe (1749–1832)
“All of women’s aspirations—whether for education, work, or any form of self-determination—ultimately rest on their ability to decide whether and when to bear children. For this reason, reproductive freedom has always been the most popular item in each of the successive feminist agendas—and the most heavily assaulted target of each backlash.”
—Susan Faludi (20th century)
“I’ll never forget my father’s response when I told him I wanted to be a lawyer. He said, “If you do this, no man will ever want you.””
—Cassandra Dunn (b. c. 1931)
“It takes twenty or so years before a mother can know with any certainty how effective her theories have been—and even then there are surprises. The daily newspapers raise the most frightening questions of all for a mother of sons: Could my once sweet babes ever become violent men? Are my sons really who I think they are?”
—Mary Kay Blakely (20th century)