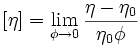Intrinsic Viscosity

Intrinsic viscosity is a measure of a solute's contribution to the viscosity of a solution. Intrinsic viscosity is frequently referred to as "Inherent Viscosity" in macromolecular literature. It is defined as

\left = \lim_{\phi \rightarrow 0} \frac{\eta - \eta_{0}}{\eta_{0}\phi}

where is the viscosity in the absence of the solute and is the volume fraction of the solute in the solution. As defined here, the intrinsic viscosity is a dimensionless number. When the solute particles are rigid spheres, the intrinsic viscosity equals \frac{5}{2}, as shown first by Albert Einstein.

In practical settings, φ is usually solute mass concentration, and the units of intrinsic viscosity are deciliters per gram (dL/g), otherwise known as inverse concentration.

Read more about Intrinsic Viscosity:  Formulae For Rigid Spheroids, General Ellipsoidal Formulae, Frequency Dependence, Applications

Famous quotes containing the word intrinsic:

    “The truth is that literature, particularly fiction, is not the pure medium we sometimes assume it to be. Response to it is affected by things other than its own intrinsic quality; by a curiosity or lack of it about the people it deals with, their outlook, their way of life.”
    —Vance Palmer (1885–1959)