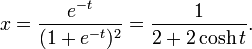Shape
The prototypical Hubbert curve is a probability density function of a logistic distribution curve. It is not a gaussian function (which is used to plot normal distributions), but the two have a similar appearance. The density of a Hubbert curve approaches zero more slowly than a gaussian function:
The graph of a Hubbert curve consists of three key elements:
- a gradual rise from zero resource production that then increases quickly
- a "Hubbert peak", representing the maximum production level
- a drop from the peak that then follows a steep production decline.
The actual shape of a graph of real world production trends is determined by various factors, such as development of enhanced production techniques, availability of competing resources, and government regulations on production or consumption. Because of such factors, real world Hubbert curves are often not symmetrical.
Read more about this topic: Hubbert Curve
Famous quotes containing the word shape:
“And yonder in the gymnasts’ garden thrives
The self-sown, self-begotten shape that gives
Athenian intellect its mastery,
Even the grey-leaved olive-tree
Miracle-bred out of the living stone....”
—William Butler Yeats (1865–1939)
“I know that each stage is not going to last forever. I used to think that when he was little. Whenever he was in a bad stage I thought that he was going to be like that for the rest of his life and that I’d better do something to shape him up. When he was in a good state, I thought he was going to be a perfect child and I would never have to worry; he was always going to stay that way.”
—Anonymous Parent of An Eight-Year-Old. As quoted in Between Generations by Ellen Galinsky, ch. 4 (1981)
“After that it came to my door. Now it lives here.
And of course: it is a soft sound, soft as a seal’s ear,
that was caught between a shape and a shape and then returned to me.”
—Anne Sexton (1928–1974)