Givens Rotation - Matrix Representation

Matrix Representation

A Givens rotation is represented by a matrix of the form

G(i, j, \theta) = \begin{bmatrix} 1 & \cdots & 0 & \cdots & 0 & \cdots & 0 \\ \vdots & \ddots & \vdots & & \vdots & & \vdots \\ 0 & \cdots & c & \cdots & -s & \cdots & 0 \\ \vdots & & \vdots & \ddots & \vdots & & \vdots \\ 0 & \cdots & s & \cdots & c & \cdots & 0 \\ \vdots & & \vdots & & \vdots & \ddots & \vdots \\ 0 & \cdots & 0 & \cdots & 0 & \cdots & 1 \end{bmatrix}

where c = cos(θ) and s = sin(θ) appear at the intersections ith and jth rows and columns. That is, the non-zero elements of Givens matrix is given by:

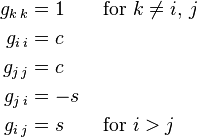\begin{align} g_{k\, k} &{}= 1 \qquad \text{for} \ k \ne i,\,j\\ g_{i\, i} &{}= c \\ g_{j\, j} &{}= c \\ g_{j\, i} &{}= -s \\ g_{i\, j} &{}= s \qquad \text{for} \ i > j \end{align} (sign of sine switches for j > i)

The product G(i,j,θ)x represents a counterclockwise rotation of the vector x in the (i,j) plane of θ radians, hence the name Givens rotation.

The main use of Givens rotations in numerical linear algebra is to introduce zeros in vectors or matrices. This effect can, for example, be employed for computing the QR decomposition of a matrix. One advantage over Householder transformations is that they can easily be parallelised, and another is that often for very sparse matrices they have a lower operation count.

Read more about this topic:  Givens Rotation

Famous quotes containing the word matrix:

    “In all cultures, the family imprints its members with selfhood. Human experience of identity has two elements; a sense of belonging and a sense of being separate. The laboratory in which these ingredients are mixed and dispensed is the family, the matrix of identity.”
    —Salvador Minuchin (20th century)