Effective Atomic Number - For A Compound or Mixture

For A Compound or Mixture

An alternative definition of the effective atomic number is one quite different to that described above. The atomic number of a material exhibits a strong and fundamental relationship with the nature of radiation interactions within that medium. There are numerous mathematical descriptions of different interaction processes that are dependent on the atomic number, Z. When dealing with composite media (i.e. a bulk material composed of more than one element), one therefore encounters the difficulty of defining Z. An effective atomic number in this context is equivalent to the atomic number but is used for compounds (e.g. water) and mixtures of different materials (such as tissue and bone). This is of most interest in terms of radiation interaction with composite materials. For bulk interaction properties, it can be useful to define an effective atomic number for a composite medium and, depending on the context, this may be done in different ways. Such methods include (i) a simple mass-weighted average, (ii) a power-law type method with some (very approximate) relationship to radiation interaction properties or (iii) methods involving calculation based on interaction cross sections. The latter is the most accurate approach (Taylor 2012), and the other more simplified approaches are often inaccurate even when used in a relative fashion for comparing materials.

In many textbooks and scientific publications, the following - simplistic and often dubious - sort of method is employed. One such proposed formula for the effective atomic number, Z_eff, is as follows:

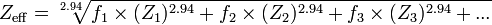
where

is the fraction of the total number of electrons associated with each element, and

is the atomic number of each element.

An example is that of water (H₂O), made up of two hydrogen atoms (Z=1) and one oxygen atom (Z=8), the total number of electrons is 1+1+8 = 10, so the fraction of electrons for the two hydrogens is (2/10) and for the one oxygen is (8/10). So the Z_eff for water is:

The effective atomic number is important for predicting how photons interact with a substance, as certain types of photon interactions depend on the atomic number. The exact formula, as well as the exponent 2.94, can depend on the energy range being used. As such, readers are reminded that this approach is of very limited applicability and may be quite misleading.

This 'power law' method, while commonly employed, is of questionable appropriateness in contemporary scientific applications within the context of radiation interactions in heterogeneous media. This approach dates back to the late 1930s when photon sources were restricted to low-energy x-ray units (Mayneord 1937). The exponent of 2.94 relates to an empirical formula for the photoelectric process which incorporates a ‘constant’ of 2.64 x 10-26, which is in fact not a constant but rather a function of the photon energy. A linear relationship between Z2.94 has been shown for a limited number of compounds for low-energy x-rays, but within the same publication it is shown that many compounds do not lie on the same trendline (Spiers et al. 1946). As such, for polyenergetic photon sources (in particular, for applications such as radiotherapy), the effective atomic number varies significantly with energy (Taylor et al. 2008). As shown by Taylor et al. (2008), it is possible to obtain a much more accurate single-valued Z_eff by weighting against the spectrum of the source. The effective atomic number for electron interactions may be calculated with a similar approach; see for instance Taylor et al. 2009 and Taylor 2011. The cross-section based approach for determining Z_eff is obviously much more complicated than the simple power-law approach described above, and this is why freely-available software has been developed for such calculations (Taylor et al. 2012).