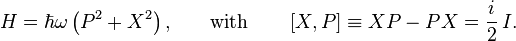Coherent States - Quantum Mechanical Definition

Quantum Mechanical Definition

Mathematically, the coherent state is defined to be the right eigenstate of the annihilation operator . Formally, this reads:

Since is not hermitian, is a complex number that is not necessarily real, and can be represented as

where is a real number. Here and are called the amplitude and phase of the state, respectively. The state is called a canonical coherent state in the literature, since there are many other types of coherent states, as can be seen in the companion article Coherent states in mathematical physics.

Physically, this formula means that a coherent state is left unchanged by the detection (or annihilation) of field excitation or, say, a particle. The eigenstate of the annihilation operator has a Poissonian number distribution (as shown below). A Poisson distribution is a necessary and sufficient condition that all detections are statistically independent. Compare this to a single-particle state ( Fock state): once one particle is detected, there is zero probability of detecting another.

The derivation of this will make use of dimensionless operators, and, usually called field quadratures in quantum optics. These operators are related to the position and momentum of a mass on a spring with constant :

For an optical field,

~E_{\rm R} = \left(\frac{\hbar\omega}{2\epsilon_0 V} \right)^{1/2} \!\!\!\cos(\theta) X~

and ~E_{\rm I} = \left(\frac{\hbar\omega}{2\epsilon_0 V}\right)^{1/2} \!\!\!\sin(\theta) X~

are the real and imaginary components of the mode of the electric field.

With these (dimensionless!) operators, the Hamiltonian of either system becomes

{H}=\hbar \omega \left({P}^{2}+{X}^{2} \right)\text{,} \qquad\text{with}\qquad \left\equiv {XP}-{PX}=\frac{i}{2}\,{I}.

Erwin Schrödinger was searching for the most classical-like states when he first introduced minimum uncertainty Gaussian wave-packets. The quantum state of the harmonic oscillator that minimizes the uncertainty relation with uncertainty equally distributed between and satisfies the equation

.

It is an eigenstate of the operator . (If the uncertainty is not balanced between and, the state is now called a squeezed coherent state.)

Schrödinger found minimum uncertainty states for the linear harmonic oscillator to be the eigenstates of, and using the notation for multi-photon states, Glauber found the state of complete coherence to all orders in the electromagnetic field to be the right eigenstate of the annihilation operator—formally, in a mathematical sense, the same state. The name coherent state took hold after Glauber's work.

The coherent state's location in the complex plane (phase space) is centered at the position and momentum of a classical oscillator of the same phase and amplitude (or the same complex electric field value for an electromagnetic wave). As shown in Figure 5, the uncertainty, equally spread in all directions, is represented by a disk with diameter 1/2. As the phase increases the coherent state circles the origin and the disk neither distorts nor spreads. This is the most similar a quantum state can be to a single point in phase space.

Since the uncertainty (and hence measurement noise) stays constant at 1/2 as the amplitude of the oscillation increases, the state behaves more and more like a sinusoidal wave, as shown in Figure 1. And, since the vacuum state is just the coherent state with, all coherent states have the same uncertainty as the vacuum. Therefore one can interpret the quantum noise of a coherent state as being due to the vacuum fluctuations.

The notation does not refer to a Fock state. For example, at, one should not mistake as a single-photon Fock state—it represents a Poisson distribution of fixed number states with a mean photon number of unity.

The formal solution of the eigenvalue equation is the vacuum state displaced to a location in phase space, i.e., it is obtained by letting the unitary displacement operator operate on the vacuum:

,

where and . This can be easily seen, as can virtually all results involving coherent states, using the representation of the coherent state in the basis of Fock states:

|\alpha\rangle =e^{-{|\alpha|^2\over2}}\sum_{n=0}^{\infty}{\alpha^n\over\sqrt{n!}}|n\rangle =e^{-{|\alpha|^2\over2}}e^{\alpha\hat a^\dagger}|0\rangle .

where are energy (number) eigenvectors of the Hamiltonian . For the corresponding Poissonian distribution, the probability of detecting photons is:

Similarly, the average photon number in a coherent state is and the variance is ~(\Delta n)^2={\rm Var}\left(\hat a^\dagger \hat a\right)= |\alpha|^2~.

In the limit of large α these detection statistics are equivalent to that of a classical stable wave for all (large) values of . These results apply to detection results at a single detector and thus relate to first order coherence (see degree of coherence). However, for measurements correlating detections at multiple detectors, higher-order coherence is involved (e.g., intensity correlations, second order coherence, at two detectors). Glauber's definition of quantum coherence involves nth-order correlation functions (n-th order coherence) for all n. The perfect coherent state has all n-orders of correlation equal to 1 (coherent). It is perfectly coherent to all orders.

Roy J. Glauber's work was prompted by the results of Hanbury-Brown and Twiss that produced long-range (hundreds or thousands of miles) first-order interference patterns through the use of intensity fluctuations (lack of second order coherence), with narrow band filters (partial first order coherence) at each detector. (One can imagine, over very short durations, a near-instantaneous interference pattern from the two detectors, due to the narrow band filters, that dances around randomly due to the shifting relative phase difference. With a coincidence counter, the dancing interference pattern would be stronger at times of increased intensity, and that pattern would be stronger than the background noise.) Almost all of optics had been concerned with first order coherence. The Hanbury-Brown and Twiss results prompted Glauber to look at higher order coherence, and he came up with a complete quantum-theoretic description of coherence to all orders in the electromagnetic field (and a quantum-theoretic description of signal-plus-noise). He coined the term coherent state and showed that they are produced when a classical electrical current interacts with the electromagnetic field.

At, from Figure 5, simple geometry gives . From this we can see that there is a tradeoff between number uncertainty and phase uncertainty, which sometimes can be interpreted as the number-phase uncertainty relation. This is not a formal uncertainty relation: there is no uniquely defined phase operator in quantum mechanics

Read more about this topic:  Coherent States

Famous quotes containing the words quantum, mechanical and/or definition:

    “A personality is an indefinite quantum of traits which is subject to constant flux, change, and growth from the birth of the individual in the world to his death. A character, on the other hand, is a fixed and definite quantum of traits which, though it may be interpreted with slight differences from age to age and actor to actor, is nevertheless in its essentials forever fixed.”
    —Hubert C. Heffner (1901–1985)

    “A man should have a farm or a mechanical craft for his culture. We must have a basis for our higher accomplishments, our delicate entertainments of poetry and philosophy, in the work of our hands.”
    —Ralph Waldo Emerson (1803–1882)

    “The definition of good prose is proper words in their proper places; of good verse, the most proper words in their proper places. The propriety is in either case relative. The words in prose ought to express the intended meaning, and no more; if they attract attention to themselves, it is, in general, a fault.”
    —Samuel Taylor Coleridge (1772–1834)