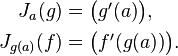Chain Rule - The Chain Rule in Higher Dimensions

The Chain Rule in Higher Dimensions

The simplest generalization of the chain rule to higher dimensions uses the total derivative. The total derivative is a linear transformation that captures how the function changes in all directions. Let f : Rm → Rk and g : Rn → Rm be differentiable functions, and let D be the total derivative operator. If a is a point in Rn, then the higher dimensional chain rule says that:

or for short,

In terms of Jacobian matrices, the rule says

That is, the Jacobian of the composite function is the product of the Jacobians of the composed functions. The higher-dimensional chain rule can be proved using a technique similar to the second proof given above.

The higher-dimensional chain rule is a generalization of the one-dimensional chain rule. If k, m, and n are 1, so that f : R → R and g : R → R, then the Jacobian matrices of f and g are 1 × 1. Specifically, they are:

\begin{align} J_a(g) &= \begin{pmatrix} g'(a) \end{pmatrix}, \\ J_{g(a)}(f) &= \begin{pmatrix} f'(g(a)) \end{pmatrix}. \end{align}

The Jacobian of f ∘ g is the product of these 1 × 1 matrices, so it is f′(g(a))g′(a), as expected from the one-dimensional chain rule. In the language of linear transformations, Da(g) is the function which scales a vector by a factor of g′(a) and Dg(a)(f) is the function which scales a vector by a factor of f′(g(a)). The chain rule says that the composite of these two linear transformations is the linear transformation Da(f ∘ g), and therefore it is the function that scales a vector by f′(g(a))g′(a).

Another way of writing the chain rule is used when f and g are expressed in terms of their components as y = f(u) = (f1(u), ..., fk(u)) and u = g(x) = (g1(x), ..., gm(x)). In this case, the above rule for Jacobian matrices is usually written as:

The chain rule for total derivatives implies a chain rule for partial derivatives. Recall that when the total derivative exists, the partial derivative in the ith coordinate direction is found by multiplying the Jacobian matrix by the ith basis vector. By doing this to the formula above, we find:

Since the entries of the Jacobian matrix are partial derivatives, we may simplify the above formula to get:

More conceptually, this rule expresses the fact that a change in the xi direction may change all of g1 through gk, and any of these changes may affect f.

In the special case where k = 1, so that f is a real-valued function, then this formula simplifies even further:

Read more about this topic:  Chain Rule

Famous quotes containing the words chain, rule, higher and/or dimensions:

    “How have I been able to live so long outside Nature without identifying myself with it? Everything lives, moves, everything corresponds; the magnetic rays, emanating either from myself or from others, cross the limitless chain of created things unimpeded; it is a transparent network that covers the world, and its slender threads communicate themselves by degrees to the planets and stars. Captive now upon earth, I commune with the chorus of the stars who share in my joys and sorrows.”
    —Gérard De Nerval (1808–1855)

    “While Michael Angelo’s Sistine roof,
    His “Morning” and his “Night” disclose
    How sinew that has been pulled tight,
    Or it may be loosened in repose,
    Can rule by supernatural right
    Yet be but sinew.”
    —William Butler Yeats (1865–1939)

    “The word which gives the key to the national vice is waste. And people who are wasteful are not wise, neither can they remain young and vigorous. In order to transmute energy to higher and more subtle levels one must first conserve it.”
    —Henry Miller (1891–1980)

    “I was surprised by Joe’s asking me how far it was to the Moosehorn. He was pretty well acquainted with this stream, but he had noticed that I was curious about distances, and had several maps. He and Indians generally, with whom I have talked, are not able to describe dimensions or distances in our measures with any accuracy. He could tell, perhaps, at what time we should arrive, but not how far it was.”
    —Henry David Thoreau (1817–1862)