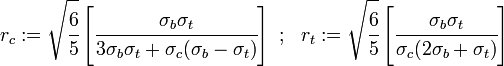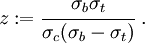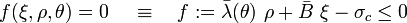Willam-Warnke Yield Criterion - Willam-Warnke Yield Function

Willam-Warnke Yield Function

In the original paper, the three-parameter Willam-Warnke yield function was expressed as

$f := \cfrac{1}{3z}~\cfrac{I_1}{\sigma_c} + \sqrt{\cfrac{2}{5}}~\cfrac{1}{r(\theta)}\cfrac{\sqrt{J_2}}{\sigma_c} - 1 \le 0$

where is the first invariant of the stress tensor, is the second invariant of the deviatoric part of the stress tensor, is the yield stress in uniaxial compression, and is the Lode angle given by

$\theta = \tfrac{1}{3}\cos^{-1}\left(\cfrac{3\sqrt{3}}{2}~\cfrac{J_3}{J_2^{3/2}}\right) ~.$

The locus of the boundary of the stress surface in the deviatoric stress plane is expressed in polar coordinates by the quantity which is given by

$r(\theta) := \cfrac{u(\theta)+v(\theta)}{w(\theta)}$

where

$\begin{align} u(\theta) := & 2~r_c~(r_c^2-r_t^2)~\cos\theta \\ v(\theta) := & r_c~(2~r_t - r_c)\sqrt{4~(r_c^2 - r_t^2)~\cos^2\theta + 5~r_t^2 - 4~r_t~r_c} \\ w(\theta) := & 4(r_c^2 - r_t^2)\cos^2\theta + (r_c-2~r_t)^2 \end{align}$

The quantities and describe the position vectors at the locations and can be expressed in terms of as

$r_c := \sqrt{\cfrac{6}{5}}\left ~;~~ r_t := \sqrt{\cfrac{6}{5}}\left$

The parameter in the model is given by

$z := \cfrac{\sigma_b\sigma_t}{\sigma_c(\sigma_b-\sigma_t)} ~.$

The Haigh-Westergaard representation of the Willam-Warnke yield condition can be written as

$f(\xi, \rho, \theta) = 0 \, \quad \equiv \quad f := \bar{\lambda}(\theta)~\rho + \bar{B}~\xi - \sigma_c \le 0$

where

$\bar{B} := \cfrac{1}{\sqrt{3}~z} ~;~~ \bar{\lambda} := \cfrac{1}{\sqrt{5}~r(\theta)} ~.$

Read more about this topic: Willam-Warnke Yield Criterion

Famous quotes containing the words yield and/or function:

“The face of nature and civilization in this our country is to a certain point a very sufficient literary field. But it will yield its secrets only to a really grasping imagination.... To write well and worthily of American things one need even more than elsewhere to be a master.”
—Henry James (1843–1916)

“It is the function of vice to keep virtue within reasonable bounds.”
—Samuel Butler (1835–1902)