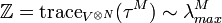Vertex Model - Discussion

Discussion

For a given state, the Boltzmann weight can be written in terms of the product of the Boltzmann weights of the corresponding vertices

where the Boltzmann weights for the vertices are written

.

The probability of the system being in any given state at a particular time, and hence the properties of the system are determined by the partition function, for which an analytic solution is desired.

where β=1/kT, T is temperature and k is Boltzmann's constant. The probability that the system is in any given microstate is given by

so that the average value of the energy of the system is given by

\langle \varepsilon \rangle = \frac{\sum_\mbox{states} \varepsilon \exp(-\beta \varepsilon)}{\sum_\mbox{states} \exp(-\beta \varepsilon)} = kT^2 \frac{\partial}{\partial T} \ln \mathbb{Z}

In order to evaluate the partition function, firstly examine the states of a row of vertices.

The external edges are free variables, with summation over the internal bonds. Hence, form the row partition function

T_{i_1 k_1 \dots k_N}^{i'_1 \ell_1 \dots l_N} = \sum_{r_1,\dots,r_{N-1}} R_{i_1 k_1}^{r_1 \ell_1} R_{r_1 k_2}^{r_2 \ell_2} \cdots R_{r_{N-1} k_N}^{i'_1 \ell_N}

This can be reformulated in terms of an auxiliary n-dimensional vector space V, with a basis, and as

and as

thereby implying that T can be written as

where the indices indicate the factors of the tensor product on which R operates. Summing over the states of the bonds in the first row with the periodic boundary conditions, gives

where is the row-transfer matrix.

By summing the contributions over two rows, the result is

which upon summation over the vertical bonds connecting the first two rows gives:

for M rows, this gives

and then applying the periodic boundary conditions to the vertical columns, the partition function can be expressed in terms of the transfer matrix as

\mathbb{Z}= \operatorname{trace}_{V^{\otimes N}}(\tau^M) \sim \lambda_{max}^M

where is the largest eigenvalue of . The approximation follows from the fact that the eigenvalues of are the eigenvalues of to the power of M, and as, the power of the largest eigenvalue becomes much larger than the others. As the trace is the sum of the eigenvalues, the problem of calculating reduces to the problem of finding the maximum eigenvalue of . This in it itself is another field of study. However, a standard approach to the problem of finding the largest eigenvalue of is to find a large family of operators which commute with . This implies that the eigenspaces are common, and restricts the possible space of solutions. Such a family of commuting operators is usually found by means of the Yang-Baxter equation, which thus relates statistical mechanics to the study of quantum groups.

Read more about this topic:  Vertex Model

Famous quotes containing the word discussion:

    “Opinions are formed in a process of open discussion and public debate, and where no opportunity for the forming of opinions exists, there may be moods—moods of the masses and moods of individuals, the latter no less fickle and unreliable than the former—but no opinion.”
    —Hannah Arendt (1906–1975)

    “Americans, unhappily, have the most remarkable ability to alchemize all bitter truths into an innocuous but piquant confection and to transform their moral contradictions, or public discussion of such contradictions, into a proud decoration, such as are given for heroism on the battle field.”
    —James Baldwin (1924–1987)

    “We cannot set aside an hour for discussion with our children and hope that it will be a time of deep encounter. The special moments of intimacy are more likely to happen while baking a cake together, or playing hide and seek, or just sitting in the waiting room of the orthodontist.”
    —Neil Kurshan (20th century)