Classification
In knot theory, the trefoil is the first nontrivial knot, and is the only knot with crossing number three. It is a prime knot, and is listed as 31 in the Alexander-Briggs notation. The Dowker notation for the trefoil is 4 6 2, and the Conway notation for the trefoil is .
The trefoil can be described as the (2,3)-torus knot. It is also the knot obtained by closing the braid σ13.
The trefoil is an alternating knot. However, it is not a slice knot, meaning that it does not bound a smooth 2-dimensional disk in the 4-dimensional ball; one way to prove this is to note that its signature is not zero. Another proof is that its Alexander polynomial does not satisfy the Fox-Milnor condition.
The trefoil is a fibered knot, meaning that its complement in is a fiber bundle over the circle . In the model of the trefoil as the set of pairs of complex numbers such that and, this fiber bundle has the Milnor map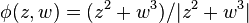 
as its fibration, and a once-punctured torus as its fiber surface. Since the knot complement is Seifert fibred with boundary, it has a horizontal incompressible surface -- this is also the fiber of the Milnor map.
Read more about this topic: Trefoil Knot