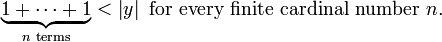Transfer Principle - Statement

Statement

The ordered field *R of nonstandard real numbers properly includes the real field R. Like all ordered fields that properly include R, this field is non-Archimedean. It means that some members x ≠ 0 of *R are infinitesimal, i.e.,

The only infinitesimal in R is 0. Some other members of *R, the reciprocals y of the nonzero infinitesimals, are infinite, i.e.,

The underlying set of the field *R is the image of R under a mapping A ↦ *A from subsets A of R to subsets of *R. In every case

with equality if and only if A is finite. Sets of the form *A for some are called standard subsets of *R. The standard sets belong to a much larger class of subsets of *R called internal sets. Similarly each function

extends to a function

these are called standard functions, and belong to the much larger class of internal functions. Sets and functions that are not internal are external.

The importance of these concepts stems from their role in the following proposition and is illustrated by the examples that follow it.

The transfer principle:

• Suppose a proposition that is true of *R can be expressed via functions of finitely many variables (e.g. (x, y) ↦ x + y), relations among finitely many variables (e.g. x ≤ y), finitary logical connectives such as and, or, not, if...then..., and the quantifiers

For example, one such proposition is

Such a proposition is true in R if and only if it is true in *R when the quantifier

replaces

and similarly for .

• Suppose a proposition otherwise expressible as simply as those considered above mentions some particular sets . Such a proposition is true in R if and only if it is true in *R with each such "A" replaced by the corresponding *A. Here are two examples:
  • The set

must be

including not only members of R between 0 and 1 inclusive, but also members of *R between 0 and 1 that differ from those by infinitesimals. To see this, observe that the sentence

is true in R, and apply the transfer principle.

• • The set *N must have no upper bound in *R (since the sentence expressing the non-existence of an upper bound of N in R is simple enough for the transfer principle to apply to it) and must contain n + 1 if it contains n, but must not contain anything between n and n + 1. Members of

are "infinite integers".)

• Suppose a proposition otherwise expressible as simply as those considered above contains the quantifier

Such a proposition is true in R if and only if it is true in *R after the changes specified above and the replacement of the quantifiers with

and