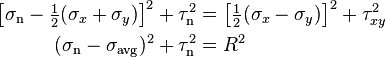Stress Transformation in Plane Stress and Plane Strain
Consider a point in a continuum under a state of plane stress, or plane strain, with stress components and all other stress components equal to zero (Figure 7.1, Figure 8.1). From static equilibrium of an infinitesimal material element at (Figure 8.2), the normal stress and the shear stress on any plane perpendicular to the - plane passing through with a unit vector making an angle of with the horizontal, i.e. is the direction cosine in the direction, is given by:
These equations indicate that in a plane stress or plane strain condition, one can determine the stress components at a point on all directions, i.e. as a function of, if one knows the stress components on any two perpendicular directions at that point. It is important to remember that we are considering a unit area of the infinitesimal element in the direction parallel to the - plane.
The principal directions (Figure 8.3), i.e. orientation of the planes where the shear stress components are zero, can be obtained by making the previous equation for the shear stress equal to zero. Thus we have:
and we obtain
This equation defines two values which are apart (Figure 8.3). The same result can be obtained by finding the angle which makes the normal stress a maximum, i.e.
The principal stresses and, or minimum and maximum normal stresses and, respectively, can then be obtained by replacing both values of into the previous equation for . This can be achieved by rearranging the equations for and, first transposing the first term in the first equation and squaring both sides of each of the equations then adding them. Thus we have
where
which is the equation of a circle of radius centered at a point with coordinates, called Mohr's circle. But knowing that for the principal stresses the shear stress, then we obtain from this equation:
When the infinitesimal element is oriented in the direction of the principal planes, thus the stresses acting on the rectangular element are principal stresses: and . Then the normal stress and shear stress as a function of the principal stresses can be determined by making . Thus we have
Then the maximum shear stress occurs when, i.e. (Figure 8.3):
Then the minimum shear stress occurs when, i.e. (Figure 8.3):
Read more about this topic: Stress Analysis
Famous quotes containing the words stress, plane and/or strain:
“It is not stressful circumstances, as such, that do harm to children. Rather, it is the quality of their interpersonal relationships and their transactions with the wider social and material environment that lead to behavioral, emotional, and physical health problems. If stress matters, it is in terms of how it influences the relationships that are important to the child.”
—Felton Earls (20th century)
“Even though I had let them choose their own socks since babyhood, I was only beginning to learn to trust their adult judgment.. . . I had a sensation very much like the moment in an airplane when you realize that even if you stop holding the plane up by gripping the arms of your seat until your knuckles show white, the plane will stay up by itself. . . . To detach myself from my children . . . I had to achieve a condition which might be called loving objectivity.”
—Anonymous Parent of Adult Children. Ourselves and Our Children, by Boston Women’s Health Book Collective, ch. 5 (1978)
“The real stumbling-block of totalitarian régimes is not the spiritual need of men for freedom of thought; it is men’s inability to stand the physical and nervous strain of a permanent state of excitement, except during a few years of their youth.”
—Simone Weil (1909–1943)