Quantum Harmonic Oscillator - N-dimensional Harmonic Oscillator | <i>N< I>-dimensional Harmonic Oscillator

N-dimensional Harmonic Oscillator

The one-dimensional harmonic oscillator is readily generalizable to N dimensions, where N = 1, 2, 3, ... . In one dimension, the position of the particle was specified by a single coordinate, x. In N dimensions, this is replaced by N position coordinates, which we label x₁, ..., x_N. Corresponding to each position coordinate is a momentum; we label these p₁, ..., p_N. The canonical commutation relations between these operators are

$\begin{matrix} \left &=& i\hbar\delta_{i,j} \\ \left &=& 0 \\ \left &=& 0 \end{matrix}$ .

The Hamiltonian for this system is

As the form of this Hamiltonian makes clear, the N-dimensional harmonic oscillator is exactly analogous to N independent one-dimensional harmonic oscillators with the same mass and spring constant. In this case, the quantities x₁, ..., x_N would refer to the positions of each of the N particles. This is a convenient property of the potential, which allows the potential energy to be separated into terms depending on one coordinate each.

This observation makes the solution straightforward. For a particular set of quantum numbers {n} the energy eigenfunctions for the N-dimensional oscillator are expressed in terms of the 1-dimensional eigenfunctions as:

$\langle \mathbf{x}|\psi_{\{n\}}\rangle =\prod_{i=1}^N\langle x_i|\psi_{n_i}\rangle$

In the ladder operator method, we define N sets of ladder operators,

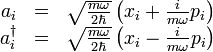$\begin{matrix} a_i &=& \sqrt{m\omega \over 2\hbar} \left(x_i + {i \over m \omega} p_i \right) \\ a^{\dagger}_i &=& \sqrt{m \omega \over 2\hbar} \left( x_i - {i \over m \omega} p_i \right) \end{matrix}$ .

By a procedure analogous to the one-dimensional case, we can then show that each of the a_i and a†_i operators lower and raise the energy by ℏω respectively. The Hamiltonian is

$H = \hbar \omega \, \sum_{i=1}^N \left(a_i^\dagger \,a_i + \frac{1}{2}\right).$

This Hamiltonian is invariant under the dynamic symmetry group U(N) (the unitary group in N dimensions), defined by

$U\, a_i^\dagger \,U^\dagger = \sum_{j=1}^N a_j^\dagger\,U_{ji}\quad\hbox{for all}\quad U \in U(N),$

where is an element in the defining matrix representation of U(N).

The energy levels of the system are

$n_i = 0, 1, 2, \dots \quad (\hbox{the number of bosons in mode } i).$

As in the one-dimensional case, the energy is quantized. The ground state energy is N times the one-dimensional energy, as we would expect using the analogy to N independent one-dimensional oscillators. There is one further difference: in the one-dimensional case, each energy level corresponds to a unique quantum state. In N-dimensions, except for the ground state, the energy levels are degenerate, meaning there are several states with the same energy.

The degeneracy can be calculated relatively easily. As an example, consider the 3-dimensional case: Define n = n₁ + n₂ + n₃. All states with the same n will have the same energy. For a given n, we choose a particular n₁. Then n₂ + n₃ = n − n₁. There are n − n₁ + 1 possible groups {n₂, n₃}. n₂ can take on the values 0 to n − n₁, and for each n₂ the value of n₃ is fixed. The degree of degeneracy therefore is:

$g_n = \sum_{n_1=0}^n n - n_1 + 1 = \frac{(n+1)(n+2)}{2}$

Formula for general N and n :

$g_n = \binom{N+n-1}{n}$

The special case N = 3, given above, follows directly from this general equation. This is however, only true for distinguishable particle, or one particle in N dimensions (as dimensions are distinguishable). For the case of N bosons in a one dimension harmonic trap, the degeneracy scales as the number of ways to partition an integer using integers less than or equal to N.

$g_n = p(N_{-},E)$

This arises due to the constraint of putting N quanta into a state ket where and, which are the same constraints as in integer partition.

Read more about this topic: Quantum Harmonic Oscillator

Famous quotes containing the word harmonic:

“For decades child development experts have erroneously directed parents to sing with one voice, a unison chorus of values, politics, disciplinary and loving styles. But duets have greater harmonic possibilities and are more interesting to listen to, so long as cacophony or dissonance remains at acceptable levels.”
—Kyle D. Pruett (20th century)