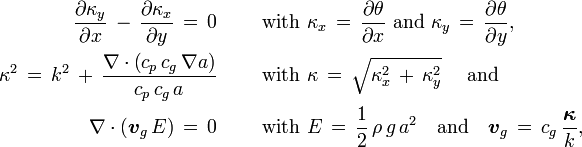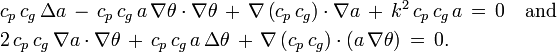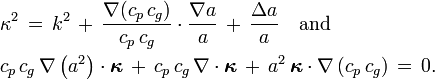Mild-slope Equation - Propagating Waves

Propagating Waves

In spatially coherent fields of propagating waves, it is useful to split the complex amplitude in its amplitude and phase, both real valued:

where

  • is the amplitude or absolute value of and
  • is the wave phase, which is the argument of

This transforms the mild-slope equation in the following set of equations (apart from locations for which is singular):

\begin{align} \frac{\partial\kappa_y}{\partial{x}}\, -\, \frac{\partial\kappa_x}{\partial{y}}\, =\, 0 \qquad &\text{ with } \kappa_x\, =\, \frac{\partial\theta}{\partial{x}} \text{ and } \kappa_y\, =\, \frac{\partial\theta}{\partial{y}}, \\ \kappa^2\, =\, k^2\, +\, \frac{\nabla\cdot\left( c_p\, c_g\, \nabla a \right)}{c_p\, c_g\, a} \qquad &\text{ with } \kappa\, =\, \sqrt{\kappa_x^2 \, +\, \kappa_y^2} \quad \text{ and} \\ \nabla \cdot \left( \boldsymbol{v}_g\, E \right)\, =\, 0 \qquad &\text{ with } E\, =\, \frac12\, \rho\, g\, a^2 \quad \text{and} \quad \boldsymbol{v}_g\, =\, c_g\, \frac{\boldsymbol{\kappa}}{k}, \end{align}

where

  • is the average wave-energy density per unit horizontal area (the sum of the kinetic and potential energy densities),
  • is the effective wavenumber vector, with components
  • is the effective group velocity vector,
  • is the fluid density, and
  • is the acceleration by the Earth's gravity.

The last equation shows that wave energy is conserved in the mild-slope equation, and that the wave energy is transported in the -direction normal to the wave crests (in this case of pure wave motion without mean currents). The effective group speed is different from the group speed

The first equation states that the effective wavenumber is irrotational, a direct consequence of the fact it is the derivative of the wave phase, a scalar field. The second equation is the eikonal equation. It shows the effects of diffraction on the effective wavenumber: only for more-or-less progressive waves, with the splitting into amplitude and phase leads to consistent-varying and meaningful fields of and . Otherwise, κ2 can even become negative. When the diffraction effects are totally neglected, the effective wavenumber κ is equal to, and the geometric optics approximation for wave refraction can be used.

Details of the derivation of the above equations

When is used in the mild-slope equation, the result is, apart from a factor :

c_p\,c_g\, \left( \Delta a\, +\, 2i\, \nabla a \cdot \nabla\theta\, -\, a\, \nabla\theta \cdot \nabla\theta\, +\, i\, a\, \Delta\theta \right)\, +\, \nabla \left( c_p\, c_g \right) \cdot \left( \nabla a\, +\, i\, a\, \nabla\theta \right)\, +\, k^2\, c_p\, c_g\, a\, =\, 0.

Now both the real part and the imaginary part of this equation have to be equal to zero:

\begin{align} & c_p\,c_g\, \Delta a\, -\, c_p\, c_g\, a\, \nabla\theta \cdot \nabla\theta\, +\, \nabla \left( c_p\, c_g \right) \cdot \nabla a\, +\, k^2\, c_p\, c_g\, a\, =\, 0 \quad \text{and} \\ & 2\, c_p\,c_g\, \nabla a \cdot \nabla\theta\, +\, c_p\, c_g\, a\, \Delta\theta\, +\, \nabla \left( c_p\, c_g \right) \cdot \left( a\, \nabla\theta \right)\, =\, 0. \end{align}

The effective wavenumber vector is defined as the gradient of the wave phase:

and its vector length is

Note that is an irrotational field, since the curl of the gradient is zero:

Now the real and imaginary parts of the transformed mild-slope equation become, first multiplying the imaginary part by :

\begin{align} &\kappa^2\, =\, k^2\, +\, \frac{\nabla(c_p\, c_g)}{c_p\, c_g} \cdot \frac{\nabla a}{a}\, +\, \frac{\Delta a}{a} \quad \text{and} \\ &c_p\, c_g\, \nabla\left(a^2\right) \cdot \boldsymbol{\kappa}\, +\, c_p\, c_g\, \nabla\cdot\boldsymbol{\kappa}\, +\, a^2\, \boldsymbol{\kappa} \cdot \nabla \left( c_p\, c_g \right)\, =\, 0. \end{align}

The first equation directly leads to the eikonal equation above for, while the second gives:

which—by noting that in which the angular frequency is a constant for time-harmonic motion—leads to the wave-energy conservation equation.

Read more about this topic:  Mild-slope Equation

Famous quotes containing the words propagating and/or waves:

    “What an infernal set of fools those schoolmarms must be! Well, if in order to please men they wish to live on air, let them. The sooner the present generation of women dies out, the better. We have idiots enough in the world now without such women propagating any more.”
    —Elizabeth Cady Stanton (1815–1902)

    “During our twenties...we act toward the new adulthood the way sociologists tell us new waves of immigrants acted on becoming Americans: we adopt the host culture’s values in an exaggerated and rigid fashion until we can rethink them and make them our own. Our idea of what adults are and what we’re supposed to be is composed of outdated childhood concepts brought forward.”
    —Roger Gould (20th century)