Liouville's Formula - Proof of Liouville's Formula

Proof of Liouville's Formula

We omit the argument x for brevity. By the Leibniz formula for determinants, the derivative of the determinant of Φ = (Φi, j )i, j ∈ {0,...,n} can be calculated by differentiating one row at a time and taking the sum, i.e.

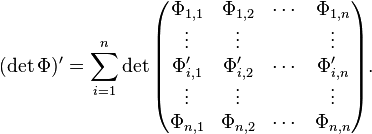
(\det\Phi)'=\sum_{i=1}^n\det\begin{pmatrix} \Phi_{1,1}&\Phi_{1,2}&\cdots&\Phi_{1,n}\\ \vdots&\vdots&&\vdots\\ \Phi'_{i,1}&\Phi'_{i,2}&\cdots&\Phi'_{i,n}\\ \vdots&\vdots&&\vdots\\ \Phi_{n,1}&\Phi_{n,2}&\cdots&\Phi_{n,n} \end{pmatrix}.

(2)

Since the matrix-valued solution Φ satisfies the equation, we have for every entry of the matrix Φ'

or for the entire row

(\Phi'_{i,1},\dots,\Phi'_{i,n}) =\sum_{j=1}^n a_{i,j}(\Phi_{j,1},\ldots,\Phi_{j,n}), \qquad i\in\{1,\ldots,n\}.

When we subtract from the i th row the linear combination

of all the other rows, then the value of the determinant remains unchanged, hence

\det\begin{pmatrix} \Phi_{1,1}&\Phi_{1,2}&\cdots&\Phi_{1,n}\\ \vdots&\vdots&&\vdots\\ \Phi'_{i,1}&\Phi'_{i,2}&\cdots&\Phi'_{i,n}\\ \vdots&\vdots&&\vdots\\ \Phi_{n,1}&\Phi_{n,2}&\cdots&\Phi_{n,n} \end{pmatrix} =\det\begin{pmatrix} \Phi_{1,1}&\Phi_{1,2}&\cdots&\Phi_{1,n}\\ \vdots&\vdots&&\vdots\\ a_{i,i}\Phi_{i,1}&a_{i,i}\Phi_{i,2}&\cdots&a_{i,i}\Phi_{i,n}\\ \vdots&\vdots&&\vdots\\ \Phi_{n,1}&\Phi_{n,2}&\cdots&\Phi_{n,n} \end{pmatrix} =a_{i,i}\det\Phi

for every i ∈ {1, . . ., n} by the linearity of the determinant with respect to every row. Hence

(3)

by (2) and the definition of the trace. It remains to show that this representation of the derivative implies Liouville's formula.

Fix x0 ∈ I. Since the trace of A is assumed to be continuous function on I, it is bounded on every closed and bounded subinterval of I and therefore integrable, hence

is a well defined function. Differentiating both sides, using the product rule, the chain rule, the derivative of the exponential function and the fundamental theorem of calculus, we obtain

due to the derivative in (3). Therefore, g has to be constant on I, because otherwise we would obtain a contradiction to the mean value theorem (applied separately to the real and imaginary part in the complex-valued case). Since g(x0) = det Φ(x0), Liouville's formula follows by solving the definition of g for det Φ(x).

Read more about this topic:  Liouville's Formula

Famous quotes containing the words proof of, proof and/or formula:

    “If we view our children as stupid, naughty, disturbed, or guilty of their misdeeds, they will learn to behold themselves as foolish, faulty, or shameful specimens of humanity. They will regard us as judges from whom they wish to hide, and they will interpret everything we say as further proof of their unworthiness. If we view them as innocent, or at least merely ignorant, they will gain understanding from their experiences, and they will continue to regard us as wise partners.”
    —Polly Berrien Berends (20th century)

    “The chief contribution of Protestantism to human thought is its massive proof that God is a bore.”
    —H.L. (Henry Lewis)

    “In the most desirable conditions, the child learns to manage anxiety by being exposed to just the right amounts of it, not much more and not much less. This optimal amount of anxiety varies with the child’s age and temperament. It may also vary with cultural values.... There is no mathematical formula for calculating exact amounts of optimal anxiety. This is why child rearing is an art and not a science.”
    —Alicia F. Lieberman (20th century)