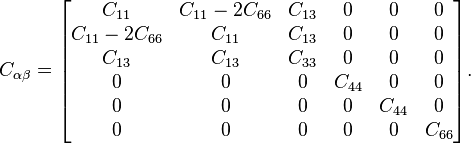Linear Elasticity - Anisotropic Homogeneous Media

Anisotropic Homogeneous Media

For anisotropic media, the stiffness tensor is more complicated. The symmetry of the stress tensor means that there are at most 6 different elements of stress. Similarly, there are at most 6 different elements of the strain tensor . Hence the 4th rank stiffness tensor may be written as a 2nd rank matrix . Voigt notation is the standard mapping for tensor indices,

$\begin{matrix} ij & =\\ \Downarrow & \\ \alpha & = \end{matrix} \begin{matrix} 11 & 22 & 33 & 23,32 & 13,31 & 12,21 \\ \Downarrow & \Downarrow & \Downarrow & \Downarrow & \Downarrow & \Downarrow & \\ 1 &2 & 3 & 4 & 5 & 6 \end{matrix}\,\!$

With this notation, one can write the elasticity matrix for any linearly elastic medium as:

$C_{ijkl} \Rightarrow C_{\alpha \beta} =\begin{bmatrix} C_{11} & C_{12} & C_{13} & C_{14} & C_{15} & C_{16} \\ C_{12} & C_{22} & C_{23} & C_{24} & C_{25} & C_{26} \\ C_{13} & C_{23} & C_{33} & C_{34} & C_{35} & C_{36} \\ C_{14} & C_{24} & C_{34} & C_{44} & C_{45} & C_{46} \\ C_{15} & C_{25} & C_{35} & C_{45} & C_{55} & C_{56} \\ C_{16} & C_{26} & C_{36} & C_{46} & C_{56} & C_{66} \end{bmatrix}. \,\!$

As shown, the matrix is symmetric, because of the linear relation between stress and strain. Hence, there are at most 21 different elements of .

The isotropic special case has 2 independent elements:

$C_{\alpha \beta} =\begin{bmatrix} K+4 \mu\ /3 & K-2 \mu\ /3 & K-2 \mu\ /3 & 0 & 0 & 0 \\ K-2 \mu\ /3 & K+4 \mu\ /3 & K-2 \mu\ /3 & 0 & 0 & 0 \\ K-2 \mu\ /3 & K-2 \mu\ /3 & K+4 \mu\ /3 & 0 & 0 & 0 \\ 0 & 0 & 0 & \mu\ & 0 & 0 \\ 0 & 0 & 0 & 0 & \mu\ & 0 \\ 0 & 0 & 0 & 0 & 0 & \mu\ \end{bmatrix}. \,\!$

The simplest anisotropic case, that of cubic symmetry has 3 independent elements:

$C_{\alpha \beta} =\begin{bmatrix} C_{11} & C_{12} & C_{12} & 0 & 0 & 0 \\ C_{12} & C_{11} & C_{12} & 0 & 0 & 0 \\ C_{12} & C_{12} & C_{11} & 0 & 0 & 0 \\ 0 & 0 & 0 & C_{44} & 0 & 0 \\ 0 & 0 & 0 & 0 & C_{44} & 0 \\ 0 & 0 & 0 & 0 & 0 & C_{44} \end{bmatrix}. \,\!$

The case of transverse isotropy, also called polar anisotropy, (with a single axis (the 3-axis) of symmetry) has 5 independent elements:

$C_{\alpha \beta} =\begin{bmatrix} C_{11} & C_{11}-2C_{66} & C_{13} & 0 & 0 & 0 \\ C_{11}-2C_{66} & C_{11} & C_{13} & 0 & 0 & 0 \\ C_{13} & C_{13} & C_{33} & 0 & 0 & 0 \\ 0 & 0 & 0 & C_{44} & 0 & 0 \\ 0 & 0 & 0 & 0 & C_{44} & 0 \\ 0 & 0 & 0 & 0 & 0 & C_{66} \end{bmatrix}. \,\!$

When the transverse isotropy is weak (i.e. close to isotropy), an alternative parametrization utilizing Thomsen parameters, is convenient for the formulas for wave speeds.

The case of orthotropy (the symmetry of a brick) has 9 independent elements:

$C_{\alpha \beta} =\begin{bmatrix} C_{11} & C_{12} & C_{13} & 0 & 0 & 0 \\ C_{12} & C_{22} & C_{23} & 0 & 0 & 0 \\ C_{13} & C_{23} & C_{33} & 0 & 0 & 0 \\ 0 & 0 & 0 & C_{44} & 0 & 0 \\ 0 & 0 & 0 & 0 & C_{55} & 0 \\ 0 & 0 & 0 & 0 & 0 & C_{66} \end{bmatrix}. \,\!$

Read more about this topic: Linear Elasticity

Famous quotes containing the words homogeneous and/or media:

“O my Brothers! love your Country. Our Country is our home, the home which God has given us, placing therein a numerous family which we love and are loved by, and with which we have a more intimate and quicker communion of feeling and thought than with others; a family which by its concentration upon a given spot, and by the homogeneous nature of its elements, is destined for a special kind of activity.”
—Giuseppe Mazzini (1805–1872)

“Today the discredit of words is very great. Most of the time the media transmit lies. In the face of an intolerable world, words appear to change very little. State power has become congenitally deaf, which is why—but the editorialists forget it—terrorists are reduced to bombs and hijacking.”
—John Berger (b. 1926)