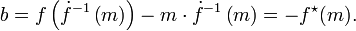Legendre Transformation - Geometric Interpretation

Geometric Interpretation

For a strictly convex function the Legendre-transformation can be interpreted as a mapping between the graph of the function and the family of tangents of the graph. (For a function of one variable, the tangents are well-defined at all but at most countably many points since a convex function is differentiable at all but at most countably many points.)

The equation of a line with slope m and y-intercept b is given by

For this line to be tangent to the graph of a function f at the point (x0, f(x0)) requires

and

f' is strictly monotone as the derivative of a strictly convex function, and the second equation can be solved for x0, allowing to eliminate x0 from the first giving the y-intercept b of the tangent as a function of its slope m:

b = f\left(\dot{f}^{-1}\left(m\right)\right) - m \cdot \dot{f}^{-1}\left(m\right) = -f^\star(m).

Here f* denotes the Legendre transform of f.

The family of tangents of the graph of f parameterized by m is therefore given by

or, written implicitly, by the solutions of the equation

The graph of the original function can be reconstructed from this family of lines as the envelope of this family by demanding

Eliminating m from these two equations gives

Identifying y with f(x) and recognizing the right side of the preceding equation as the Legendre transform of f* we find

Read more about this topic:  Legendre Transformation

Famous quotes containing the word geometric:

    “In mathematics he was greater
    Than Tycho Brahe, or Erra Pater:
    For he, by geometric scale,
    Could take the size of pots of ale;
    Resolve, by sines and tangents straight,
    If bread and butter wanted weight;
    And wisely tell what hour o’ th’ day
    The clock doth strike, by algebra.”
    —Samuel Butler (1612–1680)