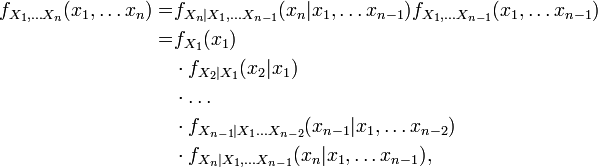Joint Probability Distribution - General Multidimensional Distributions

General Multidimensional Distributions

Remember that the cumulative distribution function for a vector of random variables is defined in terms of their joint probability distribution;


The joint distribution for two random variables can be extended to many random variables X1, ... Xn by adding them sequentially with the identity

\begin{align} f_{X_1, \ldots X_n}(x_1, \ldots x_n) =& f_{X_n | X_1, \ldots X_{n-1}}( x_n | x_1, \ldots x_{n-1}) f_{X_1, \ldots X_{n-1}}( x_1, \ldots x_{n-1} )\\ =& f_{X_1} (x_1) \\ & \cdot f_{X_2|X_1} (x_2|x_1)\\ & \cdot \dots \\ & \cdot f_{X_{n-1}| X_1 \ldots X_{n-2}}(x_{n-1}| x_1, \ldots x_{n-2} ) \\ & \cdot f_{X_n | X_1, \ldots X_{n-1}}( x_n | x_1, \ldots x_{n-1}),\end{align}

where

\begin{align} f_{X_i| X_1, \ldots X_{i-1}}(x_i | x_1, \ldots x_{i-1})= &\frac{f_{X_1, \dots X_i}(x_1,\dots x_i)}{\int f_{X_1, \dots X_i}(x_1,\dots x_{i-1},u_i) \mathrm{d} u_i}\\ = &\frac{\int \dots \int f_{X_1, \dots X_n}(x_1,\dots x_i,u_{i+1}, \dots u_n) \mathrm{d} u_{i+1}\dots \mathrm{d}u_n}{\int \dots \int \int f_{X_1, \dots X_n}(x_1,\dots x_{i-1},u_i, \dots u_n) \mathrm{d} u_i \,\mathrm{d} u_{i+1}\dots \mathrm{d}u_n} \end{align}

and

(notice, that these latter identities can be useful to generate a random variable with given distribution function ); the density of the marginal distribution is

The joint cumulative distribution function is

and the conditional distribution function is accordingly

\begin{align} F_{X_i| X_1, \ldots X_{i-1}}(x_i| x_1, \ldots x_{i-1})= &\frac{\int_{-\infty}^{x_i}f_{X_1, \dots X_i}(x_1,\dots x_{i-1},u_i)\mathrm{d}u_i}{\int_{-\infty}^\infty f_{X_1, \dots X_i}(x_1,\dots x_{i-1},u_i) \mathrm{d} u_i}\\ = &\frac{\int_{-\infty}^\infty \dots \int_{-\infty}^\infty \int_{-\infty}^{x_i} f_{X_1, \dots X_n}(x_1,\dots x_{i-1},u_i, \dots u_n) \mathrm{d} u_i\dots \mathrm{d}u_n}{\int_{-\infty}^\infty \dots \int_{-\infty}^\infty \int_{-\infty}^\infty f_{X_1, \dots X_n}(x_1,\dots x_{i-1},u_i,\dots u_n) \mathrm{d} u_i \dots \mathrm{d} u_n}. \end{align}


Expectation reads

suppose that h is smooth enough and for, then, by iterated integration by parts,

\begin{align}\mathbb{E}\left=& h(x_1,\dots x_n)+ \\ & (-1)^n \int_{-\infty}^{x_1} \dots \int_{-\infty}^{x_n} F_{X_1,\dots X_n}(u_1,\dots u_n) \frac{\partial^n}{\partial x_1 \dots \partial x_n} h(u_1,\dots u_n) \mathrm{d} u_1 \dots \mathrm{d} u_n.\end{align}

Read more about this topic:  Joint Probability Distribution

Famous quotes containing the word general:

    “Of what use, however, is a general certainty that an insect will not walk with his head hindmost, when what you need to know is the play of inward stimulus that sends him hither and thither in a network of possible paths?”
    —George Eliot [Mary Ann (or Marian)