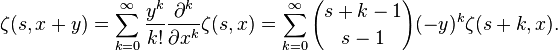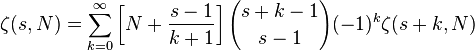Hurwitz Zeta Function - Taylor Series

Taylor Series

The derivative of the zeta in the second argument is a shift:

Thus, the Taylor series has the distinctly umbral form:

\zeta(s,x+y) = \sum_{k=0}^\infty \frac {y^k} {k!} \frac {\partial^k} {\partial x^k} \zeta (s,x) = \sum_{k=0}^\infty {s+k-1 \choose s-1} (-y)^k \zeta (s+k,x).

Closely related is the Stark–Keiper formula:

\zeta(s,N) = \sum_{k=0}^\infty \left {s+k-1 \choose s-1} (-1)^k \zeta (s+k,N)

which holds for integer N and arbitrary s. See also Faulhaber's formula for a similar relation on finite sums of powers of integers.

Read more about this topic:  Hurwitz Zeta Function

Famous quotes containing the words taylor and/or series:

    “The principle of the Gothic architecture is infinity made imaginable.”
    —Samuel Taylor Coleridge (1772–1834)

    “History is nothing but a procession of false Absolutes, a series of temples raised to pretexts, a degradation of the mind before the Improbable.”
    —E.M. Cioran (b. 1911)