Rational Values
The Hurwitz zeta function occurs in a number of striking identities at rational values. In particular, values in terms of the Euler polynomials :
and
One also has
which holds for . Here, the and are defined by means of the Legendre chi function as
and
For integer values of ν, these may be expressed in terms of the Euler polynomials. These relations may be derived by employing the functional equation together with Hurwitz's formula, given above.
Read more about this topic: Hurwitz Zeta Function
Famous quotes containing the words rational and/or values:
“... there is no such thing as a rational world and a separate irrational world, but only one world containing both.”
—Robert Musil (1880–1942)
“Everyone values the good nature of a man with a gun.”
—Mason Cooley (b. 1927)

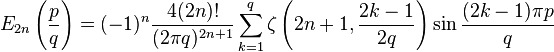
![\zeta\left(s,\frac{2p-1}{2q}\right) =
2(2q)^{s-1} \sum_{k=1}^q \left[
C_s\left(\frac{k}{q}\right) \cos \left(\frac{(2p-1)\pi k}{q}\right) +
S_s\left(\frac{k}{q}\right) \sin \left(\frac{(2p-1)\pi k}{q}\right)
\right]](http://upload.wikimedia.org/math/4/9/8/498855b49454d92f06ad65330d3b091c.png)