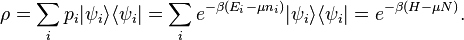Grand Canonical Ensemble - Quantum Mechanical Ensemble

Quantum Mechanical Ensemble

An ensemble of quantum mechanical systems is described by a density matrix. In a suitable representation, a density matrix ρ takes the form

where pk is the probability of a system chosen at random from the ensemble will be in the microstate

So the trace of ρ, denoted by Tr(ρ), is 1. This is the quantum mechanical analogue of the fact that the accessible region of the classical phase space has total probability 1.

It is also assumed that the ensemble in question is stationary, i.e. it does not change in time. Therefore, by Liouville's theorem, = 0, i.e. ρH = Hρ where H is the Hamiltonian of the system. Thus the density matrix describing ρ is diagonal in the energy representation.

Suppose

where Ei is the energy of the i-th energy eigenstate. If a system i-th energy eigenstate has ni number of particles, the corresponding observable, the number operator, is given by

From classical considerations, we know that the state

has (unnormalized) probability

Thus the grand canonical ensemble is the mixed state

\rho = \sum_i p_i |\psi_i \rangle \langle \psi_i| = \sum_i e^{-\beta (E_i - \mu n_i)} |\psi_i \rangle \langle \psi_i| = e^{- \beta (H - \mu N)}.

The grand partition, the normalizing constant for Tr(ρ) to be 1, is

Remember that for the grand partition, the states are states with multiple particles in Fock space, and the trace sums over all of them. In the special case of a non-interacting system, the grand partition can be simplified and expressed in terms of the eigenvalues of the single-particle Hamiltonian; after all, the eigenvalues of the multiple-particle Hamiltonian will have the form

weighting each energy state with the number of particles in that state.

Read more about this topic:  Grand Canonical Ensemble

Famous quotes containing the words quantum and/or mechanical:

    “The receipt to make a speaker, and an applauded one too, is short and easy.—Take of common sense quantum sufficit, add a little application to the rules and orders of the House, throw obvious thoughts in a new light, and make up the whole with a large quantity of purity, correctness, and elegancy of style.”
    —Philip Dormer Stanhope, 4th Earl Chesterfield (1694–1773)

    “There is only one evil, to deny life
    As Rome denied Etruria
    And mechanical America Montezuma still.”
    —D.H. (David Herbert)