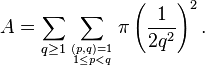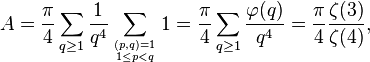Ford Circle - Total Area of Ford Circles

Total Area of Ford Circles

There is a link between the area of Ford circles, Euler's totient function φ, the Riemann zeta function ζ, and Apéry's constant ζ(3).

As no two Ford circles intersect, it follows immediately that the total area of the Ford circles

is less than 1. In fact the total area of these Ford circles is given by a convergent sum, which can be evaluated.

From the definition, the area is

A = \sum_{q\ge 1} \sum_{ (p, q)=1 \atop 1 \le p < q } \pi \left( \frac{1}{2 q^2} \right)^2.

Simplifying this expression gives

A = \frac{\pi}{4} \sum_{q\ge 1} \frac{1}{q^4} \sum_{ (p, q)=1 \atop 1 \le p < q } 1 = \frac{\pi}{4} \sum_{q\ge 1} \frac{\varphi(q)}{q^4} = \frac{\pi}{4} \frac{\zeta(3)}{\zeta(4)},

where the last equality reflects the Dirichlet generating function for Euler's totient function φ(q). Since ζ(4) = π 4/90, this finally becomes

Read more about this topic:  Ford Circle

Famous quotes containing the words total, area, ford and/or circles:

    “By sharing the information and observations with the caregiver, you have a chance to see your child through another pair of eyes. Because she has some distance and objectivity, a caregiver often sees things that a parent’s total involvement with her child doesn’t allow.”
    —Amy Laura Dombro (20th century)

    “During the Civil War the area became a refuge for service- dodging Texans, and gangs of bushwhackers, as they were called, hid in its fastnesses. Conscript details of the Confederate Army hunted the fugitives and occasional skirmishes resulted.”
    —Administration in the State of Texa, U.S. public relief program (1935-1943)

    “Love is dead; let lovers’ eyes,
    Locked in endless dreams,
    The extremes of all extremes,
    Ope no more, for now Love dies.”
    —John Ford (1586–1640?)

    “And why do you cry, my dear, why do you cry?
    It is all in the whirling circles of time.
    If millions are born millions must die,”
    —Robinson Jeffers (1887–1962)