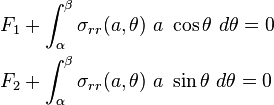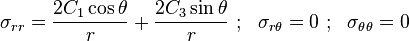Derivation of Flamant Solution
If we assume the stresses to vary as, we can pick terms containing in the stresses from Michell's solution. Then the Airy stress function can be expressed as
Therefore, from the tables in Michell's solution, we have
The constants can then, in principle, be determined from the wedge geometry and the applied boundary conditions.
However, the concentrated loads at the vertex are difficult to express in terms of traction boundary conditions because
- the unit outward normal at the vertex is undefined
- the forces are applied at a point (which has zero area) and hence the traction at that point is infinite.
To get around this problem, we consider a bounded region of the wedge and consider equilibrium of the bounded wedge. Let the bounded wedge have two traction free surfaces and a third surface in the form of an arc of a circle with radius . Along the arc of the circle, the unit outward normal is where the basis vectors are . The tractions on the arc are
Next, we examine the force and moment equilibrium in the bounded wedge and get
We require that these equations be satisfied for all values of and thereby satisfy the boundary conditions.
The traction-free boundary conditions on the edges and also imply that
except at the point .
If we assume that everywhere, then the traction-free conditions and the moment equilibrium equation are satisfied and we are left with
and along except at the point . But the field everywhere also satisfies the force equilibrium equations. Hence this must be the solution. Also, the assumption implies that .
Therefore,
To find a particular solution for we have to plug in the expression for into the force equilibrium equations to get a system of two equations which have to be solved for :
Read more about this topic: Flamant Solution
Famous quotes containing the word solution:
“Who shall forbid a wise skepticism, seeing that there is no practical question on which any thing more than an approximate solution can be had? Is not marriage an open question, when it is alleged, from the beginning of the world, that such as are in the institution wish to get out, and such as are out wish to get in?”
—Ralph Waldo Emerson (1803–1882)
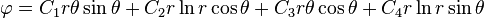


![\begin{align} \sum f_1 & = F_1 + \int_{\alpha}^{\beta} \left[\sigma_{rr}(a,\theta)~\cos\theta - \sigma_{r\theta}(a,\theta)~\sin\theta\right]~a~d\theta = 0 \\ \sum f_2 & = F_2 + \int_{\alpha}^{\beta} \left[\sigma_{rr}(a,\theta)~\sin\theta + \sigma_{r\theta}(a,\theta)~\cos\theta\right]~a~d\theta = 0 \\ \sum m_3 & = \int_{\alpha}^{\beta} \left~a~d\theta = 0 \end{align}](http://upload.wikimedia.org/math/c/6/7/c67b1d45e05b5ad20a385660875e6915.png)