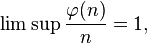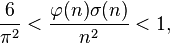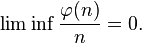Euler's Totient Function - Growth of The Function

Growth of The Function

In the words of Hardy & Wright, φ(n) is "always ‘nearly n’."

First

$\lim\sup \frac{\varphi(n)}{n}= 1,$

but as n goes to infinity, for all δ > 0

$\frac{\varphi(n)}{n^{1-\delta}}\rightarrow\infty.$

These two formulae can be proved by using little more than the formulae for φ(n) and the divisor sum function σ(n).
In fact, during the proof of the second formula, the inequality

$\frac {6}{\pi^2} < \frac{\varphi(n) \sigma(n)}{n^2} < 1,$

true for n > 1, is proven.
We also have

$\lim\inf\frac{\varphi(n)}{n}\log\log n = e^{-\gamma}.$ Here γ is Euler's constant, γ = 0.577215665..., eγ = 1.7810724..., e−γ = 0.56145948... .

Proving this, however, requires the prime number theorem. Since log log (n) goes to infinity, this formula shows that

$\lim\inf\frac{\varphi(n)}{n}= 0.$

In fact, more is true.

$\varphi(n) > \frac {n} {e^\gamma\; \log \log n + \frac {3} {\log \log n}}$ for n > 2, and

$\varphi(n) < \frac {n} {e^{ \gamma}\log \log n}$ for infinitely many n.

Concerning the second inequality, Ribenboim says "The method of proof is interesting, in that the inequality is shown first under the assumption that the Riemann hypothesis is true, secondly under the contrary assumption."

For the average order we have

$\varphi(1)+\varphi(2)+\cdots+\varphi(n) = \frac{3n^2}{\pi^2}+\mathcal{O} (n\log n)$

The "Big O" stands for a quantity that is bounded by a constant times nlog n (which is small compared to n2).

This result can be used to prove that the probability of two randomly-chosen numbers being relatively prime is

Read more about this topic: Euler's Totient Function

Famous quotes containing the words growth of the, growth of, growth and/or function:

“I conceive that the leading characteristic of the nineteenth century has been the rapid growth of the scientific spirit, the consequent application of scientific methods of investigation to all the problems with which the human mind is occupied, and the correlative rejection of traditional beliefs which have proved their incompetence to bear such investigation.”
—Thomas Henry Huxley (1825–95)

“There are enough fagots and waste wood of all kinds in the forests of most of our towns to support many fires, but which at present warm none, and, some think, hinder the growth of the young wood.”
—Henry David Thoreau (1817–1862)

“The reality is that zero defects in products plus zero pollution plus zero risk on the job is equivalent to maximum growth of government plus zero economic growth plus runaway inflation.”
—Dixie Lee Ray (b. 1924)

“The mother’s and father’s attitudes toward the child correspond to the child’s own needs.... Mother has the function of making him secure in life, father has the function of teaching him, guiding him to cope with those problems with which the particular society the child has been born into confronts him.”
—Erich Fromm (1900–1980)