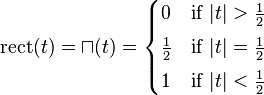Discrete-time Fourier Transform - Table of Discrete-time Fourier Transforms

Table of Discrete-time Fourier Transforms

Some common transform pairs are shown below. The following notation applies:

  • is an integer representing the discrete-time domain (in samples)
  • is a real number in, representing continuous angular frequency (in radians per sample).
    • The remainder of the transform is defined by:
  • is the discrete-time unit step function
  • is the normalized sinc function
  • is the Dirac delta function
  • is the Kronecker delta
  • is the rectangle function for arbitrary real-valued t:
\mathrm{rect}(t) = \sqcap(t) = \begin{cases} 0 & \mbox{if } |t| > \frac{1}{2} \\ \frac{1}{2} & \mbox{if } |t| = \frac{1}{2} \\ 1 & \mbox{if } |t| < \frac{1}{2} \end{cases}
  • is the triangle function for arbitrary real-valued t:
\operatorname{tri}(t) = \and (t) = \begin{cases} 1 + t; & - 1 \leq t \leq 0 \\ 1 - t; & 0 < t \leq 1 \\ 0 & \mbox{otherwise} \end{cases}
Time domain
 Frequency domain
 Remarks
integer M
integer M
The term must be interpreted as a distribution in the sense of a Cauchy principal value around its poles at .
real number a
real number a
real number a
integer M
real number a
real number W
real numbers W
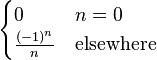\begin{cases} 0 & n=0 \\ \frac{(-1)^n}{n} & \mbox{elsewhere} \end{cases} it works as a differentiator filter
real numbers W, a
\begin{cases} 0; & n \mbox{ even} \\ \frac{2}{\pi n} ; & n \mbox{ odd} \end{cases} \begin{cases} j & \omega < 0 \\ 0 & \omega = 0 \\ -j & \omega > 0 \end{cases} Hilbert transform
real numbers A, B
complex C

Read more about this topic:  Discrete-time Fourier Transform

Famous quotes containing the words table of, table and/or transforms:

    ““A sigh for every so many breath,
    And for every so many sigh a death.
    That’s what I always tell my wife
    Is the multiplication table of life.””
    —Robert Frost (1874–1963)

    “In this sad state, God Tender Bowells run
    Out streams of Grace: And he to end all strife
    The Purest Wheate in Heaven, his deare-dear Son
    Grinds, and kneads up into this Bread of Life.
    Which Bread of Life from Heaven down came and stands
    Disht on thy Table up by Angells Hands.”
    —Edward Taylor (1645–1729)

    “Now, since our condition accommodates things to itself, and transforms them according to itself, we no longer know things in their reality; for nothing comes to us that is not altered and falsified by our Senses. When the compass, the square, and the rule are untrue, all the calculations drawn from them, all the buildings erected by their measure, are of necessity also defective and out of plumb. The uncertainty of our senses renders uncertain everything that they produce.”
    —Michel de Montaigne (1533–1592)