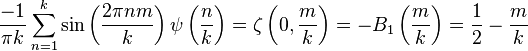Digamma Function - Gaussian Sum

Gaussian Sum

The digamma has a Gaussian sum of the form

\frac{-1}{\pi k} \sum_{n=1}^k \sin \left( \frac{2\pi nm}{k}\right) \psi \left(\frac{n}{k}\right) = \zeta\left(0,\frac{m}{k}\right) = -B_1 \left(\frac{m}{k}\right) = \frac{1}{2} - \frac{m}{k}

for integers . Here, ΞΆ(s,q) is the Hurwitz zeta function and is a Bernoulli polynomial. A special case of the multiplication theorem is

\sum_{n=1}^k \psi \left(\frac{n}{k}\right) =-k(\gamma+\log k),

and a neat generalization of this is

where q must be a natural number, but 1-qa not.

Read more about this topic:  Digamma Function

Famous quotes containing the word sum:

    “And what is the potential man, after all? Is he not the sum of all that is human? Divine, in other words?”
    —Henry Miller (1891–1980)