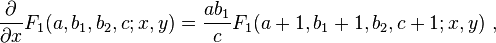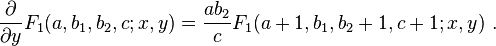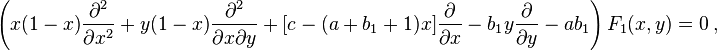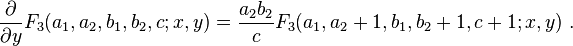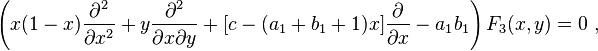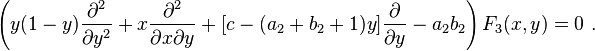Appell Series - Derivatives and Differential Equations | Derivatives Differential Equations

Derivatives and Differential Equations

For Appell's F₁, the following derivatives result from the definition by a double series:

$\frac {\partial} {\partial x} F_1(a,b_1,b_2,c; x,y) = \frac {a b_1} {c} F_1(a+1,b_1+1,b_2,c+1; x,y) ~,$

$\frac {\partial} {\partial y} F_1(a,b_1,b_2,c; x,y) = \frac {a b_2} {c} F_1(a+1,b_1,b_2+1,c+1; x,y) ~.$

From its definition, Appell's F₁ is further found to satisfy the following system of second-order differential equations:

$\left( x(1-x) \frac {\partial^2} {\partial x^2} + y(1-x) \frac {\partial^2} {\partial x \partial y} + \frac {\partial} {\partial x} - b_1 y \frac {\partial} {\partial y} - a b_1 \right) F_1(x,y) = 0 ~,$

$\left( y(1-y) \frac {\partial^2} {\partial y^2} + x(1-y) \frac {\partial^2} {\partial x \partial y} + \frac {\partial} {\partial y} - b_2 x \frac {\partial} {\partial x} - a b_2 \right) F_1(x,y) = 0 ~.$

Similarly, for F₃ the following derivatives result from the definition:

$\frac {\partial} {\partial x} F_3(a_1,a_2,b_1,b_2,c; x,y) = \frac {a_1 b_1} {c} F_3(a_1+1,a_2,b_1+1,b_2,c+1; x,y) ~,$

$\frac {\partial} {\partial y} F_3(a_1,a_2,b_1,b_2,c; x,y) = \frac {a_2 b_2} {c} F_3(a_1,a_2+1,b_1,b_2+1,c+1; x,y) ~.$

And for F₃ the following system of differential equations is obtained:

$\left( x(1-x) \frac {\partial^2} {\partial x^2} + y \frac {\partial^2} {\partial x \partial y} + \frac {\partial} {\partial x} - a_1 b_1 \right) F_3(x,y) = 0 ~,$

$\left( y(1-y) \frac {\partial^2} {\partial y^2} + x \frac {\partial^2} {\partial x \partial y} + \frac {\partial} {\partial y} - a_2 b_2 \right) F_3(x,y) = 0 ~.$

Read more about this topic: Appell Series

Famous quotes containing the word differential:

“But how is one to make a scientist understand that there is something unalterably deranged about differential calculus, quantum theory, or the obscene and so inanely liturgical ordeals of the precession of the equinoxes.”
—Antonin Artaud (1896–1948)