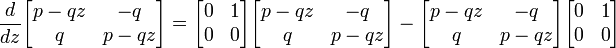Vertex Operator Algebra - A Trivial Example

A Trivial Example

To make things more concrete a trivial example of a commutative vertex algebra is presented. We take the vector space V to consist of 2-vectors, v, with components v=(p,q)T. If we think of this vector as a complex number, p+qi, the Y function can be seen as taking it from its vector form to a 2x2 matrix form of (p-qz)+qi. The p and q are real numbers.

So that multiplication between two vectors u=(p,q)T and v=(r,s)T is defined by:

The identity element IdV = (1,0)T so that:

and

and the operator T in this example is:

it is easy to check:

\frac{d}{dz} \begin{bmatrix} p-qz & -q \\ q & p-qz \end{bmatrix} = \begin{bmatrix} 0 & 1 \\ 0 & 0 \end{bmatrix} \begin{bmatrix} p-qz & -q \\ q & p-qz \end{bmatrix} - \begin{bmatrix} p-qz & -q \\ q & p-qz \end{bmatrix} \begin{bmatrix} 0 & 1 \\ 0 & 0 \end{bmatrix}

Since the Y matrices commute, locality is satisfied trivially. Non-trivial vertex algebras and vertex operator algebras would require infinite sized matrices to represent them. All the terms with powers of zn with n < 0 must be zero when applied to the identity vector IdV. i.e. of the form:

but adding a single term like this won't commute with the other terms. Hence our example would no longer be trivial since we may need an infinite number of terms to restore locality.

Read more about this topic:  Vertex Operator Algebra

Famous quotes containing the word trivial:

    “The service a man renders his friend is trivial and selfish, compared with the service he knows his friend stood in readiness to yield him, alike before he had begun to serve his friend, and now also. Compared with that good-will I bear my friend, the benefit it is in my power to render him seems small.”
    —Ralph Waldo Emerson (1803–1882)