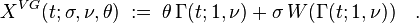Variance Gamma Process

Variance Gamma Process

In the theory of stochastic processes, a part of the mathematical theory of probability, the variance gamma process (VG), also known as Laplace motion, is a Lévy process determined by a random time change. The process has finite moments distinguishing it from many Lévy processes. There is no diffusion component in the VG process and it is thus a pure jump process. The increments are independent and follow a Variance-gamma distribution, which is a generalization of the Laplace distribution.

There are several representations of the VG process that relate it to other processes. It can for example be written as a Brownian motion with drift subjected to a random time change which follows a gamma process (equivalently one finds in literature the notation ):

X^{VG}(t; \sigma, \nu, \theta) \;:=\; \theta \,\Gamma(t; 1, \nu) + \sigma\,W(\Gamma(t; 1, \nu)) \quad.

An alternative way of stating this is that the variance gamma process is a Brownian motion subordinated to a Gamma subordinator.

Since the VG process is of finite variation it can be written as the difference of two independent gamma processes:

X^{VG}(t; \sigma, \nu, \theta) \;:=\; \Gamma(t; \mu_p, \mu_p^2\,\nu) - \Gamma(t; \mu_q, \mu_q^2\,\nu)

where

\mu_p := \frac{1}{2}\sqrt{\theta^2 + \frac{2\sigma^2}{\nu}} + \frac{\theta}{2} \quad\quad\text{and}\quad\quad \mu_q := \frac{1}{2}\sqrt{\theta^2 + \frac{2\sigma^2}{\nu}} - \frac{\theta}{2} \quad.

Alternatively it can be approximated by a compound Poisson process that leads to a representation with explicitly given (independent) jumps and their locations. This last characterization gives an understanding of the structure of the sample path with location and sizes of jumps.

On the early history of the variance-gamma process see Seneta (2000).

Read more about Variance Gamma Process:  Moments, Option Pricing, Applications To Credit Risk Modeling, Simulation

Famous quotes containing the words variance and/or process:

    “There is an untroubled harmony in everything, a full consonance in nature; only in our illusory freedom do we feel at variance with it.”
    —Fyodor Tyutchev (1803–1873)

    “At last a vision has been vouchsafed to us of our life as a whole. We see the bad with the good.... With this vision we approach new affairs. Our duty is to cleanse, to reconsider, to restore, to correct the evil without impairing the good, to purify and humanize every process of our common life, without weakening or sentimentalizing it.”
    —Woodrow Wilson (1856–1924)