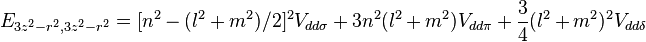Table of Interatomic Matrix Elements
In 1954 J.C. Slater and F.G. Koster published, mainly for the calculation of transition metal d-bands, a table of interatomic matrix elements
which, with a little patience and effort, can also be derived from the cubic harmonic orbitals straightforwardly. The table expresses the matrix elements as functions of LCAO two-centre bond integrals between two cubic harmonic orbitals, i and j, on adjacent atoms. The bond integrals are for example the, and for sigma, pi and delta bonds.
The interatomic vector is expressed as
where d is the distance between the atoms and l, m and n are the direction cosines to the neighboring atom.
Not all interatomic matrix elements are listed explicitly. Matrix elements that are not listed in this table can be constructed by permutation of indices and cosine directions of other matrix elements in the table.
Read more about this topic: Tight Binding
Famous quotes containing the words table of, table, matrix and/or elements:
“Remember thee?
Ay, thou poor ghost, whiles memory holds a seat
In this distracted globe. Remember thee?
Yea, from the table of my memory
I’ll wipe away all trivial fond records,
All saws of books, all forms, all pressures past
That youth and observation copied there,
And thy commandment all alone shall live
Within the book and volume of my brain,”
—William Shakespeare (1564–1616)
“The best thing about Sassy Seats is that grandmothers cannot figure out how they work and are in constant fear of the child’s falling. This often makes them forget to comment on other aspects of the child’s development, like why he is not yet talking or is still wearing diapers. Some grandmothers will spend an entire meal peering beneath the table and saying, “Is that thing steady?” rather than, “Have you had a doctor look at that left hand?””
—Anna Quindlen (20th century)
““The matrix is God?”
“In a manner of speaking, although it would be more accurate ... to say that the matrix has a God, since this being’s omniscience and omnipotence are assumed to be limited to the matrix.”
“If it has limits, it isn’t omnipotent.”
“Exactly.... Cyberspace exists, insofar as it can be said to exist, by virtue of human agency.””
—William Gibson (b. 1948)
“An illustrious individual remarks that Mrs. [Elizabeth Cady] Stanton is the salt, Anna Dickinson the pepper, and Miss [Susan B.] Anthony the vinegar of the Female Suffrage movement. The very elements get the “white male” into a nice pickle.”
—Anonymous, U.S. women’s magazine contributor. The Revolution (August 19, 1869)









![E_{xy,3z^2-r^2} = \sqrt{3} \left[ l m (n^2 - (l^2 + m^2) / 2) V_{dd\sigma} -
2 l m n^2 V_{dd\pi} + l m (1 + n^2) / 2 V_{dd\delta} \right]](http://upload.wikimedia.org/math/f/2/1/f21f69198ed239846a7af6b8fa418c6f.png)
![E_{yz,3z^2-r^2} = \sqrt{3} \left[ m n (n^2 - (l^2 + m^2) / 2) V_{dd\sigma} +
m n (l^2 + m^2 - n^2) V_{dd\pi} - m n (l^2 + m^2) / 2 V_{dd\delta} \right]](http://upload.wikimedia.org/math/4/6/9/4695e42fbc77fbb9be99fef74bcbc2e7.png)
![E_{zx,3z^2-r^2} = \sqrt{3} \left[ l n (n^2 - (l^2 + m^2) / 2) V_{dd\sigma} +
l n (l^2 + m^2 - n^2) V_{dd\pi} - l n (l^2 + m^2) / 2 V_{dd\delta} \right]](http://upload.wikimedia.org/math/2/1/e/21e7b6938bfe176c103fade410505774.png)

![E_{x^2-y^2,3z^2-r^2} = \sqrt{3} \left[
(l^2 - m^2) V_{dd\sigma} / 2 + n^2 (m^2 - l^2) V_{dd\pi} +
(1 + n^2)(l^2 - m^2) / 4 V_{dd\delta}\right]](http://upload.wikimedia.org/math/8/5/c/85c5fd7f1a498f9cb4cab7f96ad7ed08.png)