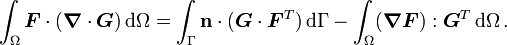Tensor Derivative (continuum Mechanics) - Integration By Parts

Integration By Parts

Another important operation related to tensor derivatives in continuum mechanics is integration by parts. The formula for integration by parts can be written as

$\int_{\Omega} \boldsymbol{F}\otimes\boldsymbol{\nabla}\boldsymbol{G}\,{\rm d}\Omega = \int_{\Gamma} \mathbf{n}\otimes(\boldsymbol{F}\otimes\boldsymbol{G})\,{\rm d}\Gamma - \int_{\Omega} \boldsymbol{G}\otimes\boldsymbol{\nabla}\boldsymbol{F}\,{\rm d}\Omega$

where and are differentiable tensor fields of arbitrary order, is the unit outward normal to the domain over which the tensor fields are defined, represents a generalized tensor product operator, and is a generalized gradient operator. When is equal to the identity tensor, we get the divergence theorem

$\int_{\Omega}\boldsymbol{\nabla}\boldsymbol{G}\,{\rm d}\Omega = \int_{\Gamma} \mathbf{n}\otimes\boldsymbol{G}\,{\rm d}\Gamma \,.$

We can express the formula for integration by parts in Cartesian index notation as

$\int_{\Omega} F_{ijk....}\,G_{lmn...,p}\,{\rm d}\Omega = \int_{\Gamma} n_p\,F_{ijk...}\,G_{lmn...}\,{\rm d}\Gamma - \int_{\Omega} G_{lmn...}\,F_{ijk...,p}\,{\rm d}\Omega \,.$

For the special case where the tensor product operation is a contraction of one index and the gradient operation is a divergence, and both and are second order tensors, we have

$\int_{\Omega} \boldsymbol{F}\cdot(\boldsymbol{\nabla}\cdot\boldsymbol{G})\,{\rm d}\Omega = \int_{\Gamma} \mathbf{n}\cdot(\boldsymbol{G}\cdot\boldsymbol{F}^T)\,{\rm d}\Gamma - \int_{\Omega} (\boldsymbol{\nabla}\boldsymbol{F}):\boldsymbol{G}^T\,{\rm d}\Omega \,.$

In index notation,

$\int_{\Omega} F_{ij}\,G_{pj,p}\,{\rm d}\Omega = \int_{\Gamma} n_p\,F_{ij}\,G_{pj}\,{\rm d}\Gamma - \int_{\Omega} G_{pj}\,F_{ij,p}\,{\rm d}\Omega \,.$

Read more about this topic: Tensor Derivative (continuum Mechanics)

Famous quotes containing the words integration and/or parts:

“The more specific idea of evolution now reached is—a change from an indefinite, incoherent homogeneity to a definite, coherent heterogeneity, accompanying the dissipation of motion and integration of matter.”
—Herbert Spencer (1820–1903)

“It is evident, from their method of propagation, that a couple of cats, in fifty years, would stock a whole kingdom; and if that religious veneration were still paid them, it would, in twenty more, not only be easier in Egypt to find a god than a man, which Petronius says was the case in some parts of Italy; but the gods must at last entirely starve the men, and leave themselves neither priests nor votaries remaining.”
—David Hume (1711–1776)