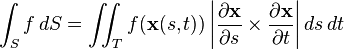Surface Integral - Surface Integrals of Scalar Fields

Surface Integrals of Scalar Fields

To find an explicit formula for the surface integral, we need to parameterize the surface of interest, S, by considering a system of curvilinear coordinates on S, like the latitude and longitude on a sphere. Let such a parameterization be x(s, t), where (s, t) varies in some region T in the plane. Then, the surface integral is given by

$\int_{S} f \,dS = \iint_{T} f(\mathbf{x}(s, t)) \left|{\partial \mathbf{x} \over \partial s}\times {\partial \mathbf{x} \over \partial t}\right| ds\, dt$

where the expression between bars on the right-hand side is the magnitude of the cross product of the partial derivatives of x(s, t), and is known as the surface element.

For example, if we want to find the surface area of some general scalar function, say, we have

$A = \int_S \,dS = \iint_T \left\|{\partial \mathbf{r} \over \partial x}\times {\partial \mathbf{r} \over \partial y}\right\| dx\, dy$

where . So that, and . So,

$\begin{align} A &{} = \iint_T \left\|\left(1, 0, {\partial f \over \partial x}\right)\times \left(0, 1, {\partial f \over \partial y}\right)\right\| dx\, dy \\ &{} = \iint_T \left\|\left(-{\partial f \over \partial x}, -{\partial f \over \partial y}, 1\right)\right\| dx\, dy \\ &{} = \iint_T \sqrt{\left({\partial f \over \partial x}\right)^2+\left({\partial f \over \partial y}\right)^2+1}\, \, dx\, dy \end{align}$

which is the familiar formula we get for the surface area of a general functional shape. One can recognize the vector in the second line above as the normal vector to the surface.

Note that because of the presence of the cross product, the above formulas only work for surfaces embedded in three-dimensional space.

Read more about this topic: Surface Integral

Famous quotes containing the words surface and/or fields:

“We say justly that the weak person is flat, for, like all flat substances, he does not stand in the direction of his strength, that is, on his edge, but affords a convenient surface to put upon. He slides all the way through life.... But the brave man is a perfect sphere, which cannot fall on its flat side and is equally strong every way.”
—Henry David Thoreau (1817–1862)

“What doubts, what hypotheses, what labyrinths of amusement, what fields of disputation, what an ocean of false learning, may be avoided by that single notion of immaterialism!”
—George Berkeley (1685–1753)