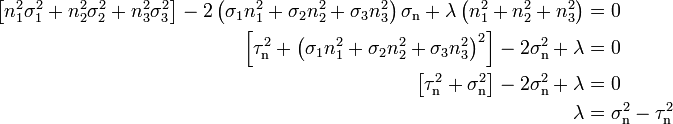Maximum and Minimum Shear Stresses
The maximum shear stress or maximum principal shear stress is equal to one-half the difference between the largest and smallest principal stresses, and acts on the plane that bisects the angle between the directions of the largest and smallest principal stresses, i.e. the plane of the maximum shear stress is oriented from the principal stress planes. The maximum shear stress is expressed as
Assuming then
The normal stress component acting on the plane for the maximum shear stress is non-zero and it is equal to
-
Derivation of the maximum and minimum shear stresses The normal stress can be written in terms of principal stresses as Knowing that, the shear stress in terms of principal stresses components is expressed as
The maximum shear stress at a point in a continuum body is determined by maximizing subject to the condition that
This is a constrained maximization problem, which can be solved using the Lagrangian multiplier technique to convert the problem into an unconstrained optimization problem. Thus, the stationary values (maximum and minimum values)of occur where the gradient of is parallel to the gradient of .
The Lagrangian function for this problem can be written as
where is the Lagrangian multiplier (which is different from the use to denote eigenvalues).
The extreme values of these functions are
thence
These three equations together with the condition may be solved for and
By multiplying the first three equations by and, respectively, and knowing that we obtain
Adding these three equations we get
this result can be substituted into each of the first three equations to obtain
Doing the same for the other two equations we have
A first approach to solve these last three equations is to consider the trivial solution . However this options does not fulfill the constrain .
Considering the solution where and, it is determine from the condition that, then from the original equation for it is seen that . The other two possible values for can be obtained similarly by assuming
- and
- and
Thus, one set of solutions for these four equations is:
These correspond to minimum values for and verifies that there are no shear stresses on planes normal to the principal directions of stress, as shown previously.
A second set of solutions is obtained by assuming and . Thus we have
To find the values for and we first add these two equations
Knowing that for
and
we have
and solving for we have
Then solving for we have
and
The other two possible values for can be obtained similarly by assuming
- and
- and
Therefore the second set of solutions for, representing a maximum for is
Therefore, assuming, the maximum shear stress is expressed by
and it can be stated as being equal to one-half the difference between the largest and smallest principal stresses, acting on the plane that bisects the angle between the directions of the largest and smallest principal stresses.
Read more about this topic: Stress (mechanics)
Famous quotes containing the words maximum, minimum and/or stresses:
“I had a quick grasp of the secret to sanity—it had become the ability to hold the maximum of impossible combinations in one’s mind.”
—Norman Mailer (b. 1923)
“There are ... two minimum conditions necessary and sufficient for the existence of a legal system. On the one hand those rules of behavior which are valid according to the system’s ultimate criteria of validity must be generally obeyed, and on the other hand, its rules of recognition specifying the criteria of legal validity and its rules of change and adjudication must be effectively accepted as common public standards of official behavior by its officials.”
—H.L.A. (Herbert Lionel Adolphus)
“Europe has a press that stresses opinions; America a press, radio, and television that emphasize news.”
—James Reston (b. 1909)