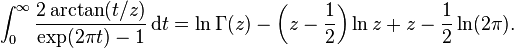Stirling's Approximation - A Convergent Version of Stirling's Formula

A Convergent Version of Stirling's Formula

Thomas Bayes showed, in a letter to John Canton published by the Royal Society in 1763, that Stirling's formula did not give a convergent series.

Obtaining a convergent version of Stirling's formula entails evaluating

$\int_0^\infty \frac{2\arctan (t/z)}{\exp(2 \pi t)-1}\,{\rm d}t = \ln\Gamma (z) - \left( z-\frac12 \right) \ln z +z - \frac12\ln(2\pi).$

One way to do this is by means of a convergent series of inverted rising exponentials. If ; then

$\int_0^\infty \frac{2\arctan (t/z)}{\exp(2 \pi t)-1} \,{\rm d}t = \sum_{n=1}^\infty \frac{c_n}{(z+1)^{\bar n}}$

where

where s(n, k) denotes the Stirling numbers of the first kind. From this we obtain a version of Stirling's series

$\begin{align} \ln \Gamma (z) & = \left( z-\frac12 \right) \ln z -z + \frac{\ln (2 \pi)}{2} \\ & {} + \frac{1}{12(z+1)} + \frac{1}{12(z+1)(z+2)} + \frac{59}{360(z+1)(z+2)(z+3)} \\ & {} + \frac{29}{60(z+1)(z+2)(z+3)(z+4)} + \cdots \end{align}$

which converges when .

Read more about this topic: Stirling's Approximation

Famous quotes containing the words version, stirling and/or formula:

“I should think that an ordinary copy of the King James version would have been good enough for those Congressmen.”
—Calvin Coolidge (1872–1933)

“Oh, if thy pride did not our joys control,
What world of loving wonders shouldst thou see!
For if I saw thee once transformed in me,
Then in thy bosom I would pour my soul;”
—William Alexander, Earl O Stirling (1580?–1640)

“I take it that what all men are really after is some form or perhaps only some formula of peace.”
—Joseph Conrad (1857–1924)