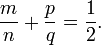Conjugate Chains
- Conjugate Steiner chains with ''n'' = 4
-
Steiner chain with the two given circles shown in red and blue.
-
Same set of circles, but with a different choice of given circles.
-
Same set of circles, but with yet another choice of given circles.
If a Steiner chain has an even number of circles, then any two diametrically opposite circles in the chain can be taken as the two given circles of a new Steiner chain to which the original circles belong. If the original Steiner chain has n circles in m wraps, and the new chain has p circles in q wraps, then the equation holds
A simple example occurs for Steiner chains of four circles (n = 4) and one wrap (m = 1). In this case, the given circles and the Steiner-chain circles are equivalent in that both types of circles are tangent to four others; more generally, Steiner-chain circles are tangent to four circles, but the two given circles are tangent to n circles. In this case, any pair of opposite members of the Steiner chain may be selected as the given circles of another Steiner chain that involves the original given circles. Since m = p = 1 and n = q = 4, Steiner's equation is satisfied:
Read more about this topic: Steiner Chain
Famous quotes containing the word chains:
“Of all men living [priests] are our greatest enemies. If it were possible, they would extinguish the very light of nature, turn the world into a dungeon, and keep mankind for ever in chains and darkness.”
—George Berkeley (1685–1753)