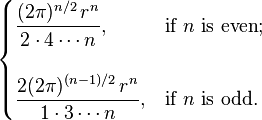Sphere - Generalization To Other Dimensions

Generalization To Other Dimensions

Spheres can be generalized to spaces of any dimension. For any natural number n, an "n-sphere," often written as Sn, is the set of points in (n + 1)-dimensional Euclidean space which are at a fixed distance r from a central point of that space, where r is, as before, a positive real number. In particular:

  • a 0-sphere is a pair of endpoints of an interval (−r, r) of the real line
  • a 1-sphere is a circle of radius r
  • a 2-sphere is an ordinary sphere
  • a 3-sphere is a sphere in 4-dimensional Euclidean space.

Spheres for n > 2 are sometimes called hyperspheres.

The n-sphere of unit radius centered at the origin is denoted Sn and is often referred to as "the" n-sphere. Note that the ordinary sphere is a 2-sphere, because it is a 2-dimensional surface (which is embedded in 3-dimensional space).

The surface area of the (n − 1)-sphere of radius 1 is

where Γ(z) is Euler's Gamma function.

Another expression for the surface area is

\begin{cases} \displaystyle \frac{(2\pi)^{n/2}\,r^{n-1}}{2 \cdot 4 \cdots (n-2)}, & \text{if } n \text{ is even}; \\ \\ \displaystyle \frac{2(2\pi)^{(n-1)/2}\,r^{n-1}}{1 \cdot 3 \cdots (n-2)}, & \text{if } n \text{ is odd}. \end{cases}

and the volume is the surface area times or

\begin{cases} \displaystyle \frac{(2\pi)^{n/2}\,r^n}{2 \cdot 4 \cdots n}, & \text{if } n \text{ is even}; \\ \\ \displaystyle \frac{2(2\pi)^{(n-1)/2}\,r^n}{1 \cdot 3 \cdots n}, & \text{if } n \text{ is odd}. \end{cases}

Read more about this topic:  Sphere

Famous quotes containing the word dimensions:

    “Is it true or false that Belfast is north of London? That the galaxy is the shape of a fried egg? That Beethoven was a drunkard? That Wellington won the battle of Waterloo? There are various degrees and dimensions of success in making statements: the statements fit the facts always more or less loosely, in different ways on different occasions for different intents and purposes.”
    —J.L. (John Langshaw)