Special Linear Lie Algebra - Representation Theory Of

Representation Theory Of

The simplest non-trivial Lie algebra is, consisting of two by two matrices with zero trace. There are three basis elements, ,, and, with

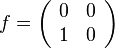and

The commutators are

, and

Let be a finite irreducible representation of, and let be an eigenvector of with the highest eigenvalue . Then,

or

Since is the eigenvector of highest eigenvalue, . Similarly, we can show that

and since h has a lowest eigenvalue, there is a such that . We will take the smallest such that this happens.

We can then recursively calculate

and we find

Taking, we get

Since we chose to be the smallest exponent such that, we conclude that . From this, we see that

, ...

are all nonzero, and it is easy to show that they are linearly independent. Therefore, for each, there is a unique, up to isomorphism, irreducible representation of dimension spanned by elements, ... .

The beautiful special case of shows a general way to find irreducible representations of Lie Algebras. Namely, we divide the algebra to three subalgebras "h" (the Cartan Subalgebra), "e", and "f", which behave approximately like their namesakes in . Namely, in a irreducible representation, we have a "highest" eigenvector of "h", on which "e" acts by zero. The basis of the irreducible representation is generated by the action of "f" on the highest eigenvectors of "h".