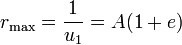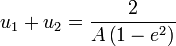Schwarzschild Geodesics - Precession of Orbits

Precession of Orbits

The function sn and its square sn2 have periods of 4K and 2K, respectively, where K is defined by the equation

$K = \int_{0}^{1} \frac{dy}{\sqrt{\left( 1 - y^{2} \right) \left( 1 - k^{2} y^{2} \right) }}$

Therefore, the change in φ over one oscillation of u (or, equivalently, one oscillation of r) equals

$\Delta \varphi = \frac{4K}{\sqrt{r_{s} \left( u_{3} - u_{1} \right) }}$

In the classical limit, u₃ approaches 1/r_s and is much larger than u₁ or u₂. Hence, k2 is approximately

$k^{2} = \frac{u_{2} - u_{1}}{u_{3} - u_{1}} \approx r_{s} \left( u_{2} - u_{1} \right) \ll 1$

For the same reasons, the denominator of Δφ is approximately

$\frac{1}{\sqrt{r_{s} \left( u_{3} - u_{1} \right) }} = \frac{1}{\sqrt{1 - r_{s} \left(2 u_{1} + u_{2} \right)}} \approx 1 + \frac{1}{2} r_{s} \left( 2u_{1} + u_{2} \right)$

Since the modulus k is close to zero, the period K can be expanded in powers of k; to lowest order, this expansion yields

$K \approx \int_{0}^{1} \frac{dy}{\sqrt{1 - y^{2} }} \left( 1 + \frac{1}{2} k^{2} y^{2} \right) = \frac{\pi}{2} \left( 1 + \frac{k^{2}}{4} \right)$

Substituting these approximations into the formula for Δφ yields a formula for angular advance per radial oscillation

$\delta \varphi = \Delta \varphi - 2\pi \approx \frac{3}{2} \pi r_{s} \left( u_{1} + u_{2} \right)$

For an elliptical orbit, u₁ and u₂ represent the inverses of the longest and shortest distances, respectively. These can be expressed in terms of the ellipse's semiaxis A and its eccentricity e,

$r_{\mathrm{max}} = \frac{1}{u_{1}} = A (1 + e)$

$r_{\mathrm{min}} = \frac{1}{u_{2}} = A (1 - e)$

giving

$u_{1} + u_{2} = \frac{2}{A \left( 1 - e^{2} \right)}$

Substituting the definition of r_s gives the final equation

$\delta \varphi \approx \frac{6\pi G M}{c^{2} A \left( 1 - e^{2} \right)}$

Read more about this topic: Schwarzschild Geodesics

Famous quotes containing the words precession of, precession and/or orbits:

“But how is one to make a scientist understand that there is something unalterably deranged about differential calculus, quantum theory, or the obscene and so inanely liturgical ordeals of the precession of the equinoxes.”
—Antonin Artaud (1896–1948)

“But how is one to make a scientist understand that there is something unalterably deranged about differential calculus, quantum theory, or the obscene and so inanely liturgical ordeals of the precession of the equinoxes.”
—Antonin Artaud (1896–1948)

“To me, however, the question of the times resolved itself into a practical question of the conduct of life. How shall I live? We are incompetent to solve the times. Our geometry cannot span the huge orbits of the prevailing ideas, behold their return, and reconcile their opposition. We can only obey our own polarity.”
—Ralph Waldo Emerson (1803–1882)