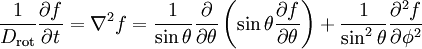Rotational Diffusion - Rotational Version of Fick's Law

Rotational Version of Fick's Law

A rotational version of Fick's law of diffusion can be defined. Let each rotating molecule be associated with a vector n of unit length n·n=1; for example, n might represent the orientation of an electric or magnetic dipole moment. Let f(θ, φ, t) represent the probability density distribution for the orientation of n at time t. Here, θ and φ represent the spherical angles, with θ being the polar angle between n and the z-axis and φ being the azimuthal angle of n in the x-y plane. The rotational version of Fick's law states

$\frac{1}{D_{\mathrm{rot}}} \frac{\partial f}{\partial t} = \nabla^{2} f = \frac{1}{\sin\theta} \frac{\partial}{\partial \theta}\left( \sin\theta \frac{\partial f}{\partial \theta} \right) + \frac{1}{\sin^{2} \theta} \frac{\partial^{2} f}{\partial \phi^{2}}$

This partial differential equation (PDE) may be solved by expanding f(θ, φ, t) in spherical harmonics for which the mathematical identity holds

$\frac{1}{\sin\theta} \frac{\partial}{\partial \theta}\left( \sin\theta \frac{\partial Y^{m}_{l}}{\partial \theta} \right) + \frac{1}{\sin^{2} \theta} \frac{\partial^{2} Y^{m}_{l}}{\partial \phi^{2}} = -l(l+1) Y^{m}_{l}$

Thus, the solution of the PDE may be written

$f(\theta, \phi, t) = \sum_{l=0}^{\infty} \sum_{m=-l}^{l} C_{lm} Y^{m}_{l}(\theta, \phi) e^{-t/\tau_{l}}$

where C_lm are constants fitted to the initial distribution and the time constants equal

$\tau_{l} = \frac{1}{D_{\mathrm{rot}}l(l+1)}$

Read more about this topic: Rotational Diffusion

Famous quotes containing the words version and/or law:

“I should think that an ordinary copy of the King James version would have been good enough for those Congressmen.”
—Calvin Coolidge (1872–1933)

“The law isn’t justice. It’s a very imperfect mechanism. If you press exactly the right buttons and are also lucky, justice may show up in the answer. A mechanism is all the law was ever intended to be.”
—Raymond Chandler (1888–1959)