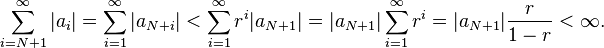Ratio Test - Proof

Proof

Below is a proof of the validity of the original ratio test.

Suppose that . We can then show that the series converges absolutely by showing that its terms will eventually become less than those of a certain convergent geometric series. To do this, let . Then r is strictly between L and 1, and for sufficiently large n (say, n greater than N). Hence for each n > N and i > 0, and so

\sum_{i=N+1}^{\infty}|a_{i}| = \sum_{i=1}^{\infty}|a_{N+i}| < \sum_{i=1}^{\infty}r^{i}|a_{N+1}| = |a_{N+1}|\sum_{i=1}^{\infty}r^{i} = |a_{N+1}|\frac{r}{1 - r} < \infty.

That is, the series converges absolutely.

On the other hand, if L > 1, then for sufficiently large n, so that the limit of the summands is non-zero. Hence the series diverges.

Read more about this topic:  Ratio Test

Famous quotes containing the word proof:

    “a meek humble Man of modest sense,
    Who preaching peace does practice continence;
    Whose pious life’s a proof he does believe,
    Mysterious truths, which no Man can conceive.”
    —John Wilmot, 2d Earl Of Rochester (1647–1680)

    “The chief contribution of Protestantism to human thought is its massive proof that God is a bore.”
    —H.L. (Henry Lewis)

    “In the reproof of chance
    Lies the true proof of men.”
    —William Shakespeare (1564–1616)