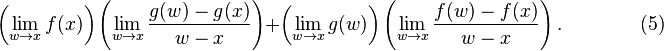Product Rule - Proof of The Product Rule

Proof of The Product Rule

A rigorous proof of the product rule can be given using the properties of limits and the definition of the derivative as a limit of Newton's difference quotient.

If

and ƒ and g are each differentiable at the fixed number x, then

Now the difference

is the area of the big rectangle minus the area of the small rectangle in the illustration.

The region between the smaller and larger rectangle can be split into two rectangles, the sum of whose areas is

Therefore the expression in (1) is equal to

Assuming that all limits used exist, (4) is equal to

\left(\lim_{w\to x}f(x)\right) \left(\lim_{w\to x} {g(w) - g(x) \over w - x}\right) + \left(\lim_{w\to x} g(w)\right) \left(\lim_{w\to x} {f(w) - f(x) \over w - x} \right). \qquad\qquad(5)

Now

This holds because f(x) remains constant as w → x.

This holds because differentiable functions are continuous (g is assumed differentiable in the statement of the product rule).

Also:

and

because f and g are differentiable at x;

We conclude that the expression in (5) is equal to

Read more about this topic:  Product Rule

Famous quotes containing the words proof of, proof, product and/or rule:

    “Ah! I have penetrated to those meadows on the morning of many a first spring day, jumping from hummock to hummock, from willow root to willow root, when the wild river valley and the woods were bathed in so pure and bright a light as would have waked the dead, if they had been slumbering in their graves, as some suppose. There needs no stronger proof of immortality. All things must live in such a light. O Death, where was thy sting? O Grave, where was thy victory, then?”
    —Henry David Thoreau (1817–1862)

    “If any proof were needed of the progress of the cause for which I have worked, it is here tonight. The presence on the stage of these college women, and in the audience of all those college girls who will some day be the nation’s greatest strength, will tell their own story to the world.”
    —Susan B. Anthony (1820–1906)

    “Everything that is beautiful and noble is the product of reason and calculation.”
    —Charles Baudelaire (1821–1867)

    “Where women are concerned, the rule is never to go out with anyone better dressed than you.”
    —John Malkovich (b. 1953)