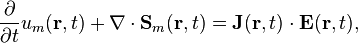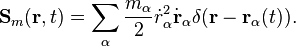Poynting's Theorem - Generalization

Generalization

The mechanical energy counterpart of the above theorem for the electromagnetic energy continuity equation is

$\frac{\partial}{\partial t} u_m(\mathbf{r},t) + \nabla\cdot \mathbf{S}_m (\mathbf{r},t) = \mathbf{J}(\mathbf{r},t)\cdot\mathbf{E}(\mathbf{r},t),$

where u_m is the (mechanical) kinetic energy density in the system. It can be described as the sum of kinetic energies of particles α (e.g., electrons in a wire), whose trajectory is given by r_α(t):

where S_m is the flux of their energies, or a "mechanical Poynting vector":

$\mathbf{S}_m (\mathbf{r},t) = \sum_{\alpha} \frac{m_{\alpha}}{2} \dot{r}^2_{\alpha}\dot{\mathbf{r}}_{\alpha} \delta(\mathbf{r}-\mathbf{r}_{\alpha}(t)).$

Both can be combined via the Lorentz force, which the electromagnetic fields exert on the moving charged particles (see above), to the following energy continuity equation or energy conservation law:

$\frac{\partial}{\partial t}\left(u_e + u_m\right) + \nabla\cdot \left( \mathbf{S}_e + \mathbf{S}_m\right) = 0,$

covering both types of energy and the conversion of one into the other.

Read more about this topic: Poynting's Theorem