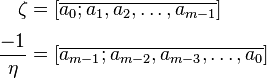Reduced Surds
The quadratic surd is said to be reduced if ζ > 1 and its conjugate satisfies the inequalities −1 < η < 0. For instance, the golden ratio φ is a reduced surd because its conjugate ½(1 −√5) is greater than −1 and less than zero. On the other hand, the square root of two is not a reduced surd because its conjugate is less than −1.
Galois proved that the regular continued fraction which represents a quadratic surd ζ is purely periodic if and only if ζ is a reduced surd. In fact, Galois showed more than this. He also proved that if ζ is a reduced quadratic surd and η is its conjugate, then the continued fractions for ζ and for (−1/η) are both purely periodic, and the repeating block in one of those continued fractions is the mirror image of the repeating block in the other. In symbols we have
where ζ is any reduced quadratic surd, and η is its conjugate.
From these two theorems of Galois a result already known to Lagrange can be deduced. If r > 1 is a rational number that is not a perfect square, then
In particular, if n is any non-square positive integer, the regular continued fraction expansion of √n contains a repeating block of length m, in which the first m − 1 partial denominators form a palindromic string.
Read more about this topic: Periodic Continued Fraction
Famous quotes containing the word reduced:
“The newspapers are the ruling power. Any other government is reduced to a few marines at Fort Independence. If a man neglects to read the Daily Times, government will go down on its knees to him, for this is the only treason these days.”
—Henry David Thoreau (1817–1862)