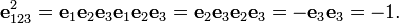The Three-dimensional Euclidean Space
The following list represents an instance of a complete basis for the space,
which forms an eight-dimensional space, where the multiple indices indicate the product of the respective basis vectors, for example
The grade of a basis element is defined in terms of the vector multiplicity, such that
| Grade | Type | Basis element/s |
|---|---|---|
| 0 | Unitary real scalar | |
| 1 | Vector | |
| 2 | Bivector | |
| 3 | Trivector volume element |
According to the fundamental axiom, two different basis vectors anticommute,
or in other words,
This means that the volume element squares to
Moreover, the volume element commutes with any other element of the algebra, so that it can be identified with the complex number, whenever there is no danger of confusion. In fact, the volume element along with the real scalar forms an algebra isomorphic to the standard complex algebra. The volume element can be used to rewrite an equivalent form of the basis as
| Grade | Type | Basis element/s |
|---|---|---|
| 0 | Unitary real scalar | |
| 1 | Vector | |
| 2 | Bivector |
|
| 3 | Trivector volume element |
Read more about this topic: Paravector
Famous quotes containing the word space:
“The true gardener then brushes over the ground with slow and gentle hand, to liberate a space for breath round some favourite; but he is not thinking about destruction except incidentally. It is only the amateur like myself who becomes obsessed and rejoices with a sadistic pleasure in weeds that are big and bad enough to pull, and at last, almost forgetting the flowers altogether, turns into a Reformer.”
—Freya Stark (1893–1993)